Mathematical expressions of the fundamental laws of conservation of the mass, the momentum and the energy of a gas, which describe the state of the gas. A gas is a population of a large number of particles (molecules, atoms, ions) in continuous chaotic motion. Computations of the interactions and of the motions of the individual particles are exceedingly difficult, and for this reason a statistical, or continuous, approach is adopted in describing the state of the gas. When this is done, the overall state of the particles of the gas is characterized by a distribution function of the particles defined in a seven-dimensional phase space 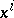 ,
, 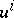 ,
, 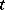 (
( ) or in the four-dimensional space
) or in the four-dimensional space 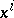 ,
, 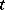 (
(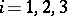 ). In the former case one considers a scalar distribution function
). In the former case one considers a scalar distribution function
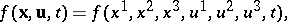 |
in which the magnitudes  are continuously-varying arguments,
are continuously-varying arguments, 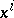 and
and 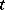 vary in bounded or unbounded intervals, and
vary in bounded or unbounded intervals, and 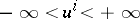 . The function
. The function 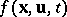 itself satisfies Boltzmann's integro-differential equation (cf. Boltzmann equation; Kinetic equation) or else satisfies other equations, depending on the physical premises (cf. Bogolyubov chain of equations; Vlasov kinetic equation). In the latter case the distribution function describing the state of the gas is a vector function
itself satisfies Boltzmann's integro-differential equation (cf. Boltzmann equation; Kinetic equation) or else satisfies other equations, depending on the physical premises (cf. Bogolyubov chain of equations; Vlasov kinetic equation). In the latter case the distribution function describing the state of the gas is a vector function
depending on four arguments 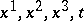 which all vary continuously and independently in the interval
which all vary continuously and independently in the interval 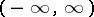 . In such a case a "particle" is to be understood, strictly speaking, as a material element of the gas which occupies an infinitesimal volume and has a certain velocity
. In such a case a "particle" is to be understood, strictly speaking, as a material element of the gas which occupies an infinitesimal volume and has a certain velocity 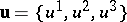 , which is a function of the arguments
, which is a function of the arguments 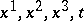 . Here
. Here  is the density of the gas, i.e. the mass of the gas per unit volume,
is the density of the gas, i.e. the mass of the gas per unit volume, 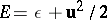 is the overall energy of the mass of the gas and
is the overall energy of the mass of the gas and  is the internal energy of a unit mass of the gas.
is the internal energy of a unit mass of the gas.
On the assumption of local thermodynamic equilibrium, the Boltzmann equation yield the laws of conservation of gas dynamics in their integral form. In an inertial orthonormal coordinate system
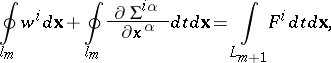 | (1) |
where  is the volume of the space bounded by the surface
is the volume of the space bounded by the surface 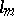 ,
, 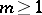 . The relations (1) are valid for an arbitrary volume
. The relations (1) are valid for an arbitrary volume 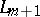 with boundary
with boundary 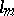 in the
in the 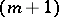 -dimensional phase space
-dimensional phase space 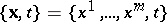 . The magnitudes
. The magnitudes 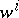 ,
, 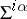 ,
, 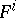 have the following form in the three-dimensional case:
have the following form in the three-dimensional case:
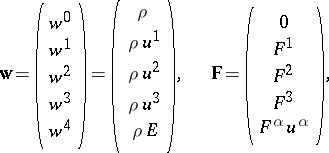 | (2) |
where 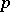 is the pressure of the gas,
is the pressure of the gas, 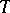 is the temperature of the gas,
is the temperature of the gas,  is the Kronecker symbol,
is the Kronecker symbol, 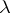 is the viscosity coefficient of compression,
is the viscosity coefficient of compression, 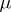 is the viscosity coefficient of motion, and
is the viscosity coefficient of motion, and 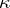 is the coefficient of thermal conduction. The notation of the formulas is that employed in tensor analysis.
is the coefficient of thermal conduction. The notation of the formulas is that employed in tensor analysis.
A system of differential equations in divergence form is employed for smooth flows:
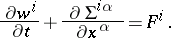 | (3) |
After the equations of state have been added, this set of equations becomes closed. At thermodynamic equilibrium the equations of state assume the form
 | (4) |
If the system is not at equilibrium, these magnitudes may depend on the gradients of the flow function.
The representation (2) has a certain physical meaning:
correspond, respectively, to convective fluxes of matter, momentum, energy; to the spherical non-dissipative part of the stress tensor, i.e. to the pressure; and to the dissipative part of the stresses (viscosity, thermal diffusion). It is used in the splitting method to obtain effective integration schemes of the problems in gas dynamics.
A gas flow can be described in various coordinate systems. In addition to the coordinate system rigidly attached to the physical space — the Galilean (or Euler) coordinate system — various mobile coordinate systems, which need not be Cartesian or Galilean, are also employed. The Lagrangian coordinate system, connected with the gas particles, is very extensively used. In this system each material element has a fixed coordinate. In Euler's method, at each moment of time 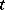 the parameters of the state of the gas are defined as functions of the coordinates
the parameters of the state of the gas are defined as functions of the coordinates 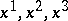 (Euler coordinates) of points of some mobile coordinate system, while the vector
(Euler coordinates) of points of some mobile coordinate system, while the vector  denotes the velocity of the gas particle which is located at the point
denotes the velocity of the gas particle which is located at the point 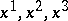 at the moment of time
at the moment of time 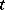 . In the Lagrange method, the velocity
. In the Lagrange method, the velocity 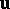 and the thermodynamic magnitudes are specified for each particle as a function of the time
and the thermodynamic magnitudes are specified for each particle as a function of the time 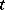 . If the gas particle is fixed with the aid of parameters
. If the gas particle is fixed with the aid of parameters 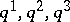 , then the flow parameters of the gas are obtained as a function of the time
, then the flow parameters of the gas are obtained as a function of the time 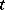 and of
and of 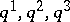 (Lagrange coordinates). The connection between the Euler and the Lagrange coordinates has the form
(Lagrange coordinates). The connection between the Euler and the Lagrange coordinates has the form
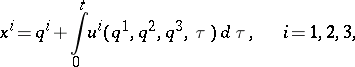 |
where 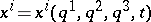 are the Euler coordinates of the particle which is located at the point
are the Euler coordinates of the particle which is located at the point 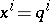 at the moment of time
at the moment of time 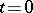 . If there is one spatial variable, and if the gas-dynamic variables are continuously-differentiable functions, then the equations of a viscous heat-conducting gas have the following form:
. If there is one spatial variable, and if the gas-dynamic variables are continuously-differentiable functions, then the equations of a viscous heat-conducting gas have the following form:
in Euler coordinates:
in Lagrange coordinates
where  ,
, 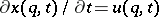 .
.
In the case of an arbitrary mobile coordinate system it is often expedient to simultaneously transform the velocity components according to the tensor law. If
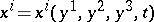 | (5) |
is a transformation of spatial coordinates under which the time coordinate remains unchanged, then the mapping (5) may be connected with the flow of the gas itself, and it will then define a field of local coordinate systems depending on this flow. More general transformations, including a change of the time coordinate, are also possible.
An important role in the theory of the equations of gas dynamics is played by the analysis of the small parameters 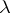 ,
, 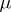 ,
, 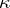 ,
, 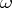 (
(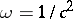 is the compressibility coefficient) forming part of (3). If
is the compressibility coefficient) forming part of (3). If 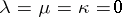 , then (3) represents the equations of the dynamics of an ideal gas; if
, then (3) represents the equations of the dynamics of an ideal gas; if 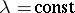 ,
,  ,
, 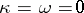 , equation (3) represents the Navier–Stokes equations of an incompressible liquid. These equations are not of Cauchy–Kovalevskaya type. If
, equation (3) represents the Navier–Stokes equations of an incompressible liquid. These equations are not of Cauchy–Kovalevskaya type. If  ,
, 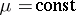 ,
, 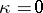 ,
, 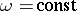 , one obtains a system of parabolic equations of Cauchy–Kovalevskaya type which is not strongly parabolic. In turbulence theory and in the theory of non-Newtonian liquids the coefficients
, one obtains a system of parabolic equations of Cauchy–Kovalevskaya type which is not strongly parabolic. In turbulence theory and in the theory of non-Newtonian liquids the coefficients 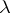 ,
, 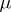 may depend on the gradients of gas-dynamic magnitudes.
may depend on the gradients of gas-dynamic magnitudes.
The defining relations (2) and, in particular, the state equations (4), characterize the type of the system of equations (3) of gas dynamics and a number of its qualitative features. Thus, for an ideal compressible gas ( ) the system of equations (3) is hyperbolic if
) the system of equations (3) is hyperbolic if
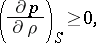 | (6) |
where the entropy 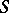 is defined by the relation (the second law of thermodynamics)
is defined by the relation (the second law of thermodynamics)
Condition (6) is local, depends on the solution, and may be violated in certain cases. Thus, in the case of the van der Waals equation of state, condition (6) may be violated, the equations become elliptic and the solution is unstable.
The conservation laws (1) make it possible to formulate a generalized solution of the equations of gas dynamics which is no longer necessarily continuous and does not satisfy the differential equations of gas dynamics (3). A complete theory of generalized equations of gas dynamics has not been developed, but the simplest generalized solutions have been thoroughly studied; these include, for example, shock waves, centred rarified waves, contact flows, etc. There exists a hypothesis according to which a generalized solution of the equations of an ideal compressible gas is the limit of the corresponding solution for the viscous gas as  and
and  . This statement has been rigorously proved for one-dimensional shock waves and certain special cases for equations of the type
. This statement has been rigorously proved for one-dimensional shock waves and certain special cases for equations of the type
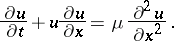 |
Of special interest are the stationary equations of gas dynamics, which are basically connected with the problems of stationary flow around bodies in an infinite space or stationary flow through channels. In such cases the solution of the system of equations (3) is independent of 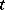 , and the system takes the form
, and the system takes the form
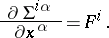 | (7) |
For the stationary system of equations (7) a certain boundary value problem, which may be very complicated, is posed, and equation (7) itself may be both of elliptic and of mixed type. For instance, in the problem of the flow of an ideal compressible gas and on the assumption that this flow is potential, the following equation is obtained in the two-dimensional case:
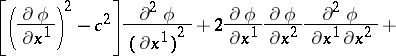 | (8) |
where  ,
, 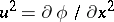 ,
, 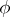 is the velocity potential, and the square of the velocity of sound
is the velocity potential, and the square of the velocity of sound 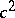 may be found from the Bernoulli integral:
may be found from the Bernoulli integral:
For equation (8) one may pose the problem of flow around a given contour 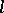 :
:
where 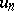 is the normal component of the velocity vector along the normal to the contour
is the normal component of the velocity vector along the normal to the contour  . If
. If 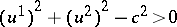 , equation (8) is hyperbolic; if
, equation (8) is hyperbolic; if 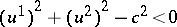 , it is elliptic. Transition from elliptic to hyperbolic type is possible (transonic flow). It can be shown that the boundary value problem is ill-posed (cf. Ill-posed problems) in the case of transonic flow, since the smallest change in the contour may render the boundary value problem unsolvable in the class of continuous functions.
, it is elliptic. Transition from elliptic to hyperbolic type is possible (transonic flow). It can be shown that the boundary value problem is ill-posed (cf. Ill-posed problems) in the case of transonic flow, since the smallest change in the contour may render the boundary value problem unsolvable in the class of continuous functions.
Of major interest are problems of gas-dynamic instability and turbulence, which are usually described in the framework of the theory of non-self-adjoint equations (Orr–Sommerfeld equation, Reynolds equation). In the context of practical applications the equations of gas dynamics which describe the motion of more complex media have become very important (multi-phase media, non-Newtonian liquids, magnetical hydrodynamics). Most equations of mathematical physics are the result of a linearization of equations of gas dynamics. For the numerical solution of problems in gas dynamics cf. Gas dynamics, numerical methods of.
References
| [1] | J. Serrin, "Mathematical principles of classical fluid mechanics" S. Flügge (ed.) , Handbuch der Physik , 8/1 , Springer (1959) pp. 125–263 MR0108115 MR0108116 MR0108117 MR0108118 |
| [2] | L.I. Sedov, "A course in continuum mechanics" , 1–4 , Wolters-Noordhoff (1971–1972) (Translated from Russian) Zbl 0308.73006 Zbl 0284.73001 Zbl 0241.76001 Zbl 0308.73005 |
| [3] | L.D. Landau, E.M. Lifshits, "Mechanics" , Pergamon (1965) (Translated from Russian) MR0183138 Zbl 0178.57901 |
| [4] | N.E. Kochin, I.A. Kibel', N.V. Roze, "Theoretical hydrodynamics" , Interscience (1964) (Translated from Russian) |
| [5] | G.G. Branover, A.M. Tsinober, "Magnetic hydrodynamics of incompressible media" , Moscow (1970) (In Russian) |
| [6] | B.L. Rozhdestvenskii, N.N. Yanenko, "Systems of quasilinear equations and their applications to gas dynamics" , Amer. Math. Soc. (1983) (Translated from Russian) MR0694243 |
References
| [a1] | R. Courant, K.O. Friedrichs, "Supersonic flow and shock waves" , Interscience (1948) MR0029615 Zbl 0041.11302 |
| [a2] | H.W. Liepmann, A. Roshko, "Elements of gas dynamics" , Wiley (1957) MR0118167 |
| [a3] | W.R. Sears (ed.) , General theory of high speed aerodynamics , Princeton Univ. Press (1954) MR0064569 Zbl 0057.18302 |
| [a4] | C. Cercignani, "The Boltzmann equation and its applications" , Springer (1988) MR1313028 Zbl 0646.76001 |
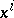 ,
, 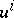 ,
, 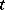 (
( ) or in the four-dimensional space
) or in the four-dimensional space 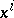 ,
, 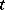 (
(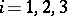 ). In the former case one considers a scalar distribution function
). In the former case one considers a scalar distribution function
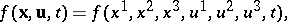
 are continuously-varying arguments,
are continuously-varying arguments, 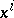 and
and 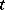 vary in bounded or unbounded intervals, and
vary in bounded or unbounded intervals, and 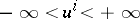 . The function
. The function 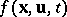 itself satisfies Boltzmann's integro-differential equation (cf. Boltzmann equation; Kinetic equation) or else satisfies other equations, depending on the physical premises (cf. Bogolyubov chain of equations; Vlasov kinetic equation). In the latter case the distribution function describing the state of the gas is a vector function
itself satisfies Boltzmann's integro-differential equation (cf. Boltzmann equation; Kinetic equation) or else satisfies other equations, depending on the physical premises (cf. Bogolyubov chain of equations; Vlasov kinetic equation). In the latter case the distribution function describing the state of the gas is a vector function
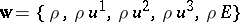
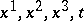 which all vary continuously and independently in the interval
which all vary continuously and independently in the interval 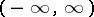 . In such a case a "particle" is to be understood, strictly speaking, as a material element of the gas which occupies an infinitesimal volume and has a certain velocity
. In such a case a "particle" is to be understood, strictly speaking, as a material element of the gas which occupies an infinitesimal volume and has a certain velocity 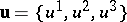 , which is a function of the arguments
, which is a function of the arguments 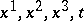 . Here
. Here  is the density of the gas, i.e. the mass of the gas per unit volume,
is the density of the gas, i.e. the mass of the gas per unit volume, 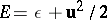 is the overall energy of the mass of the gas and
is the overall energy of the mass of the gas and  is the internal energy of a unit mass of the gas.
is the internal energy of a unit mass of the gas.
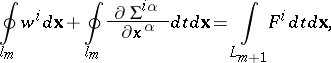
 is the volume of the space bounded by the surface
is the volume of the space bounded by the surface 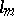 ,
, 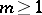 . The relations (1) are valid for an arbitrary volume
. The relations (1) are valid for an arbitrary volume 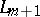 with boundary
with boundary 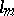 in the
in the 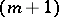 -dimensional phase space
-dimensional phase space 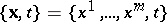 . The magnitudes
. The magnitudes 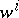 ,
, 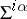 ,
, 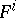 have the following form in the three-dimensional case:
have the following form in the three-dimensional case:
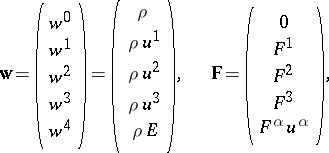
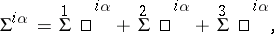

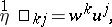


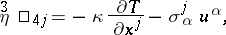
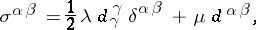
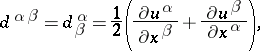
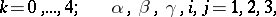
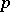 is the pressure of the gas,
is the pressure of the gas, 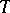 is the temperature of the gas,
is the temperature of the gas,  is the Kronecker symbol,
is the Kronecker symbol, 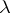 is the viscosity coefficient of compression,
is the viscosity coefficient of compression, 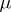 is the viscosity coefficient of motion, and
is the viscosity coefficient of motion, and 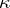 is the coefficient of thermal conduction. The notation of the formulas is that employed in tensor analysis.
is the coefficient of thermal conduction. The notation of the formulas is that employed in tensor analysis.
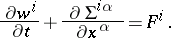


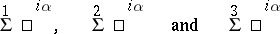
 the parameters of the state of the gas are defined as functions of the coordinates
the parameters of the state of the gas are defined as functions of the coordinates 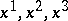 (Euler coordinates) of points of some mobile coordinate system, while the vector
(Euler coordinates) of points of some mobile coordinate system, while the vector  denotes the velocity of the gas particle which is located at the point
denotes the velocity of the gas particle which is located at the point 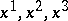 at the moment of time
at the moment of time 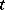 . In the Lagrange method, the velocity
. In the Lagrange method, the velocity 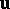 and the thermodynamic magnitudes are specified for each particle as a function of the time
and the thermodynamic magnitudes are specified for each particle as a function of the time 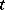 . If the gas particle is fixed with the aid of parameters
. If the gas particle is fixed with the aid of parameters 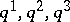 , then the flow parameters of the gas are obtained as a function of the time
, then the flow parameters of the gas are obtained as a function of the time 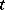 and of
and of 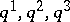 (Lagrange coordinates). The connection between the Euler and the Lagrange coordinates has the form
(Lagrange coordinates). The connection between the Euler and the Lagrange coordinates has the form
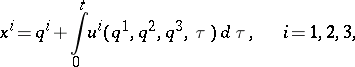
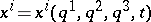 are the Euler coordinates of the particle which is located at the point
are the Euler coordinates of the particle which is located at the point 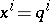 at the moment of time
at the moment of time 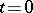 . If there is one spatial variable, and if the gas-dynamic variables are continuously-differentiable functions, then the equations of a viscous heat-conducting gas have the following form:
. If there is one spatial variable, and if the gas-dynamic variables are continuously-differentiable functions, then the equations of a viscous heat-conducting gas have the following form:
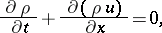
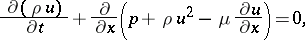
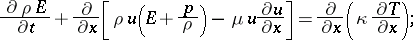

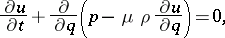
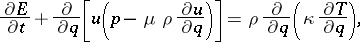
 ,
, 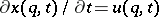 .
.
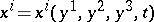
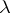 ,
, 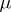 ,
, 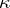 ,
, 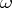 (
(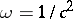 is the compressibility coefficient) forming part of (3). If
is the compressibility coefficient) forming part of (3). If 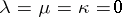 , then (3) represents the equations of the dynamics of an ideal gas; if
, then (3) represents the equations of the dynamics of an ideal gas; if 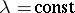 ,
,  ,
, 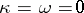 , equation (3) represents the Navier–Stokes equations of an incompressible liquid. These equations are not of Cauchy–Kovalevskaya type. If
, equation (3) represents the Navier–Stokes equations of an incompressible liquid. These equations are not of Cauchy–Kovalevskaya type. If  ,
, 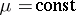 ,
, 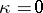 ,
, 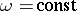 , one obtains a system of parabolic equations of Cauchy–Kovalevskaya type which is not strongly parabolic. In turbulence theory and in the theory of non-Newtonian liquids the coefficients
, one obtains a system of parabolic equations of Cauchy–Kovalevskaya type which is not strongly parabolic. In turbulence theory and in the theory of non-Newtonian liquids the coefficients 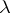 ,
, 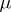 may depend on the gradients of gas-dynamic magnitudes.
may depend on the gradients of gas-dynamic magnitudes.
 ) the system of equations (3) is hyperbolic if
) the system of equations (3) is hyperbolic if
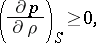
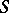 is defined by the relation (the second law of thermodynamics)
is defined by the relation (the second law of thermodynamics)
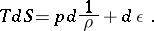
 and
and  . This statement has been rigorously proved for one-dimensional shock waves and certain special cases for equations of the type
. This statement has been rigorously proved for one-dimensional shock waves and certain special cases for equations of the type
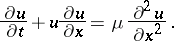
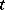 , and the system takes the form
, and the system takes the form
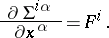
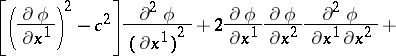
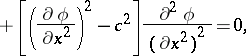
 ,
, 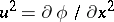 ,
, 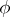 is the velocity potential, and the square of the velocity of sound
is the velocity potential, and the square of the velocity of sound 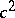 may be found from the Bernoulli integral:
may be found from the Bernoulli integral:
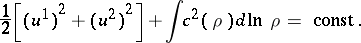
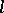 :
:

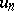 is the normal component of the velocity vector along the normal to the contour
is the normal component of the velocity vector along the normal to the contour  . If
. If 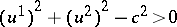 , equation (8) is hyperbolic; if
, equation (8) is hyperbolic; if 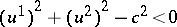 , it is elliptic. Transition from elliptic to hyperbolic type is possible (transonic flow). It can be shown that the boundary value problem is ill-posed (cf. Ill-posed problems) in the case of transonic flow, since the smallest change in the contour may render the boundary value problem unsolvable in the class of continuous functions.
, it is elliptic. Transition from elliptic to hyperbolic type is possible (transonic flow). It can be shown that the boundary value problem is ill-posed (cf. Ill-posed problems) in the case of transonic flow, since the smallest change in the contour may render the boundary value problem unsolvable in the class of continuous functions.