Fundamental domain

of a discrete group of transformations of a topological space

A subset 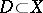containing elements from all the orbits (cf. Orbit) of , with exactly one element from orbits in general position. There are various versions of the exact definition of a fundamental domain. Sometimes a fundamental domain is any subset belonging to a given 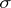-algebra (for example, the Borel 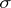-algebra) and containing exactly one representative from each orbit. However, if 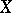is a topological manifold, then a fundamental domain is usually a subset that is the closure of an open subset and is such that the subsets , , 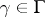have pairwise no common interior points and form a locally finite covering of 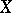. For example, as a fundamental domain of the group of parallel translations of the plane 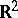by integer vectors one can take the square

The choice of a fundamental domain is, as a rule, non-unique.

Comments

The chambers of the Weyl group 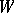are examples of fundamental domains of 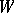in its reflection representation.