Functional calculus

A homomorphism of a certain function algebra (cf. Algebra of functions) into the algebra of continuous linear operators on a topological vector space . A functional calculus is one of the basic tools of general spectral analysis and the theory of Banach algebras and it enables one to use function-analytic methods in these disciplines. Usually, is a topological (in particular, normed) function algebra on a certain subset of the space containing the polynomials in the variables (often as a dense subset), so that a functional calculus is a natural extension of the polynomial calculus in the commuting operators , ; in this case one says that the collection 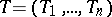admits an -calculus and one writes . 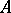An -calculus for is a kind of spectral theorem, since the correspondence , where 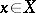, and 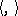is the duality between and , determines a weak operator-valued -distribution which commutes with .

The classical functional calculus of von Neumann–Murray–Dunford (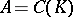, is a reflexive space) leads to the operator (projection) spectral measure

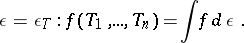The functional calculus of Riesz–Dunford (, , 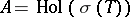that is, all functions holomorphic on the spectrum 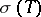of the operator 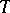) leads to the formula

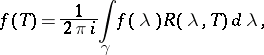where is the resolvent of and 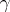is a contour enclosing inside and on which the function is regular. Formulas of the latter type with several variables (operators) depend on the notation for a linear functional on 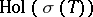and on the way the joint spectrum 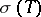of the collection 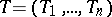is defined (the size of the functional calculus also depends on the definition of ).

If is a spectral operator, if and 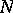are its scalar and quasi-nilpotent parts, respectively, and if 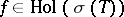, then the formula

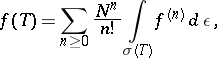where is a resolution of the identity for 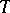, enables one to extend the Riesz–Dunford functional calculus for 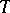to a wider class of functions. In particular, if 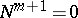, then 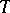admits a functional calculus on the class 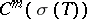of -times continuously-differentiable functions. If 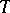is an operator of scalar type, then one can substitute bounded Borel functions on in this formula. In particular, the normal operators on a Hilbert space admit such a functional calculus. The converse is true: If an operator 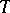admits such a functional calculus (for operators in reflexive spaces it is sufficient to assume the existence of a functional calculus on the class of continuous functions), then 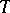is a spectral operator of scalar type (in a Hilbert space this is a linear operator that is similar to a normal operator).

In [5] the non-analytic 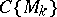-calculus was constructed for operators with a resolvent of sufficiently slow growth near the spectrum; this was based on the Carleman classes 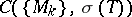(cf. Quasi-analytic class) and used the formula

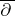where is the so-called -extension of the function 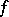across the boundary of the spectrum 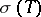, that is, a 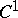-function with compact support in for which

Here

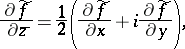and the operator satisfies

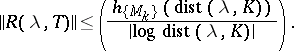On the other hand, bounds on the operator polynomials lead to more extensive calculi (than ). For example, if is a Hilbert space, then the von Neumann–Heinz inequality

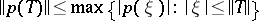leads to the Szökefalvi-Nagy–Foias functional calculus ( is the algebra of all holomorphic and bounded functions in the disc 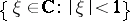, is a contraction without unitary parts), which has many applications in the theory of functional models for contraction operators. The analogue of the von Neumann–Heinz inequality for symmetric function spaces provides a functional calculus in terms of multipliers (of corresponding convolution spaces [8]).

Applications. The type of a functional calculus admitting an operator 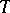is invariant under a linear similarity and can be used successfully to classify operators. In particular, there is an extensive theory of the so-called 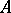-scalar operators, which can be applied to many classes of operators and is not confined to classical spectral theory. For a successful use of a functional calculus it is expedient to have the so-called spectral-mapping theorems:

Such theorems have been proved for all the functional calculi listed above (after giving a suitable meaning to the right-hand side of the formula).

If the algebra 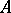contains a fine partition of unity (for example, if 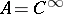), then one can construct a local spectral analysis from an 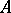-functional calculus and, in particular, one can prove the existence of non-trivial invariant subspaces of the operator 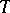(if contains more than one point); an example is an operator 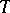(in a Banach space) with a spectrum that lies on a smooth curve and , where 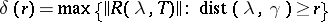. A corollary of the local analysis is Shilov's theorem on idempotents [2].

References

[1]	N. Dunford, J.T. Schwartz, "Linear operators" , 1–3 , Interscience (1958–1971)
[2]	N. Bourbaki, "Elements of mathematics. Spectral theories" , Addison-Wesley (1977) (Translated from French)
[3]	L. Waelbroeck, "Etude spectrale des algèbres complètes" Acad. Roy. Belgique Cl. Sci. , 31 : 7 (1960)
[4]	J.L. Taylor, "The analytic-functional calculus for several commuting operators" Acta Math. , 125 : 1–2 (1970) pp. 1–38
[5]	E.M. Dyn'kin, "An operator calculus based on the Cauchy–Green formula" Zap. Nauch. Sem. Leningrad. Otdel. Mat. Inst. , 30 (1972) pp. 33–39 (In Russian)
[6]	J. von Neumann, "Eine Spektraltheorie für allgemeine Operatoren eines unitären Räumes" Math. Nachr. , 4 (1950–1951) pp. 258–281
[7]	B. Szökefalvi-Nagy, Ch. Foiaş, "Harmonic analysis of operators on Hilbert space" , North-Holland (1970) (Translated from French)
[8]	V.V. Peller, "Estimates of operator polynomials in symmetric spaces. Functional calculus for absolute contraction operators" Math. Notes , 25 (1979) pp. 464–471 Mat. Zametki , 25 : 6 (1979) pp. 899–912
[9]	I. Colojoară, C. Foiaş, "Theory of generalized spectral operators" , Gordon & Breach (1968)
[10]	Yu.I. Lyubich, V.I. Matsaev, "Operators with separable spectrum" Mat. Sb. , 56 : 2 (1962) pp. 433–468 (In Russian)
[11]	J. Mikusiński, "Operational calculus" , Pergamon (1959) (Translated from Polish)
[12]	V.P. Maslov, "Operational methods" , MIR (1976) (Translated from Russian)

Comments

For a systematic treatment of analytic functional calculi in several variables cf. [a1].

References

[a1]	F.H. Vasilescu, "Analytic functional calculus and spectral decompositions" , Reidel & Ed. Academici (1982)