Fubini form
A differential form (quadratic 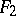 or cubic
or cubic 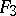 ) on which the construction of projective differential geometry is based. They were introduced by G. Fubini (see [1]).
) on which the construction of projective differential geometry is based. They were introduced by G. Fubini (see [1]).
Let 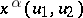 be (homogeneous) projective coordinates of a point on a surface with intrinsic coordinates
be (homogeneous) projective coordinates of a point on a surface with intrinsic coordinates 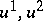 , and let
, and let
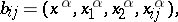 |
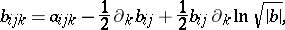 |
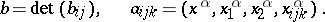 |
Then the Fubini forms are defined as follows:
 |
However, the projective coordinates themselves are not uniquely determined: they admit the introduction of arbitrary multiples and homogeneous linear transformations. Therefore the Fubini forms are defined only up to a factor, and in order to avoid difficulties connected with this one normalizes the coordinates and the forms defined in terms of them. For example, the Fubini forms preserve their value (up to sign) under unimodular projective transformations. The ratio  is called the projective line element, and is independent of the normalization (and determines the projective metric element).
is called the projective line element, and is independent of the normalization (and determines the projective metric element).
The Fubini forms that are constructed by metric means, starting from the second fundamental form and the Darboux form (defined by the Darboux tensor),
 |
are invariant under equi-affine transformations, and can therefore be used as a basis for equi-affine differential geometry.
References
| [1] | G. Fubini, E. Čech, "Geometria proettiva differenziale" , 1–2 , Zanichelli (1926–1927) |
| [2] | V.F. Kagan, "Foundations of the theory of surfaces in a tensor setting" , 2 , Moscow-Leningrad (1948) (In Russian) |
| [3] | P.A. Shirokov, A.P. Shirokov, "Differentialgeometrie" , Teubner (1962) (Translated from Russian) |
Fubini form. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Fubini_form&oldid=47002