Flat form

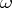A measurable -dimensional differential form on an open set 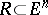such that: 1) the co-mass (cf. Mass and co-mass) 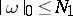for a given 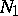; and 2) there exists an with

for any simplex 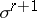satisfying the following condition: There exists a measurable 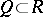, , 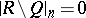such that 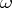is measurable on 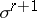and on any one of its boundaries , making up 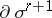; moreover,

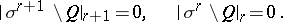Here, 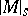denotes the -dimensional Lebesgue measure of the intersection of the set 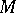with some -dimensional plane.

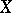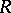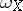If is an -dimensional flat cochain in , there exists a bounded -dimensional form in 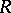which is measurable in any simplex 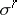with respect to the plane which contains 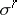, and

(1)

Also

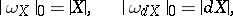where is the co-mass of the cochain . Conversely, to any -dimensional flat form 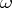in 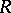there corresponds, according to formula (1), a unique -dimensional flat cochain 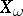for any simplex 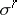which satisfies the above condition; moreover,

The form 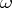and the cochain are called associated. Forms associated with the same cochain are equivalent, i.e. are equal almost-everywhere in 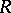, and comprise the flat representative.

There is a one-to-one correspondence between the -dimensional flat cochains and the classes of equivalent bounded measurable functions 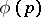, given by 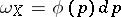, and

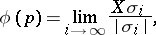where 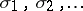is a sequence of -dimensional simplices contracting towards the point such that their diameters tend to zero, but such that

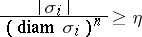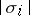for some value of , where is the volume 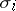for all .

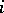Let 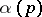be a measurable summable function in 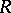whose values are -vectors; it is said to correspond to an -dimensional flat chain if

(2)

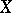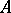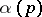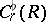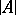for all -dimensional flat cochains ( is then called a Lebesgue chain). The mapping is a linear one-to-one mapping of the set of equivalence classes of functions into the space of flat chains ; also, , where is the mass of the chain , (cf. Mass and co-mass) and 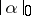is the mass of the -vector . In addition, the set of images of continuous functions is dense in .

Formulas (1) and (2) generalize similar results for sharp forms and sharp cochains (cf. Sharp form); for instance, the differential of the flat form 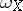, defined by the formula 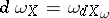, is also a flat form, and Stokes' theorem: is valid for any simplex ; an -dimensional flat cochain is the weak limit of smooth cochains, i.e. cochains for which the associated forms 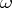are smooth, etc.

References

[1]	H. Whitney, "Geometric integration theory" , Princeton Univ. Press (1957)