First boundary value problem

A boundary value problem of special form, in which it is required to find solutions to a differential equation

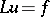(1)

of even order 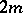in a region 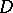of the variables for given values of the function and all its (normal) derivatives of order not exceeding 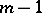on the boundary 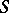of 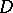(or on a part of it). These conditions are usually put in the form

(2)

where is the derivative along the outward normal to 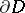. The functions , , 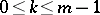are called the Dirichlet data, and the problem (1), (2) is called a Dirichlet problem if .

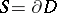For an ordinary differential equation

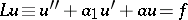(3)

in a domain of variables 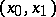, the first boundary value problem is defined by the boundary conditions

For a linear uniformly-elliptic equation

(4)

the first boundary value problem (the Dirichlet problem) consists in finding solutions to this equation subject to the condition

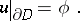If the functions 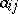, , 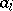, , , 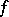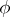and the 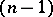-dimensional manifold 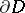are sufficiently smooth, this is a Fredholm problem. In particular, if the measure of 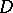is sufficiently small or if 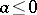in 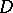, then the problem is uniquely solvable. The smoothness conditions can be weakened considerably not only with respect to the coefficients in the equations and the Dirichlet data but also with respect to the boundary .

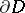If (1) is a system of 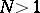equations for an unknown 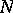-component vector , then the first boundary value problem is posed analogously. In that case there is a substantial difference between the Dirichlet problems for systems (3) and (4): while (3), (2) is always a Fredholm problem, this needs not apply to the problem (4), (2). For example, the homogeneous Dirichlet problem for the uniformly-elliptic Bitsadze system (cf. [1])

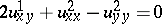in the disc 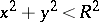has an infinite number of linearly independent solutions. This example provides a reference point for various additional conditions on 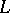(regular ellipticity, strong ellipticity) ensuring that the Dirichlet problem is of Fredholm type.

For linear parabolic equations, the first boundary value problem is posed in a cylinder, and the support for the Dirichlet data is provided by the base and the lateral surface. For example, for the thermal-conductance equation (heat equation)

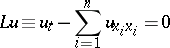the solution has to be determined in a region

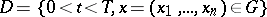and the support for the Dirichlet data 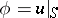is

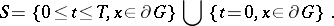If the boundary 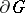is a smooth manifold of dimension 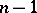, if the function 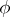is smooth and if a compatibility condition is satisfied on 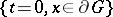, then the first boundary value problem is uniquely solvable.

References

[1]	A.V. Bitsadze, "Some classes of partial differential equations" , Gordon & Breach (1988) (Translated from Russian)
[2]	L. Bers, F. John, M. Schechter, "Partial differential equations" , Interscience (1964)
[3]	R. Courant, D. Hilbert, "Methods of mathematical physics. Partial differential equations" , 2 , Interscience (1965) (Translated from German)
[4]	C. Miranda, "Partial differential equations of elliptic type" , Springer (1970) (Translated from Italian)
[5]	I.G. Petrovskii, "Partial differential equations" , Saunders (1967) (Translated from Russian)
[6]	A.V. Bitsadze, "Equations of mathematical physics" , MIR (1980) (Translated from Russian)
[7]	L. Hörmander, "Linear partial differential operators" , Springer (1976)

Comments

The first boundary value problem has been generalized to the notion of an elliptic boundary value problem. See [a1], Chapt. 20, also for a study of the Fredholm properties.

For the theory of boundary value problems for non-linear elliptic equations, which undergoes intensive development nowadays (1988), see [a2], [a3].

References

[a1]	L.V. Hörmander, "The analysis of linear partial differential operators" , 3 , Springer (1985)
[a2]	D. Gilbarg, N.S. Trudinger, "Elliptic partial differential equations of second order" , Springer (1977)
[a3]	O.A. Ladyzhenskaya, N.N. Ural'tseva, "Linear and quasilinear elliptic equations" , Acad. Press (1968) (Translated from Russian)
[a4]	A. Friedman, "Partial differential equations" , Holt, Rinehart & Winston (1969)
[a5]	A. Friedman, "Partial differential equations of parabolic type" , Prentice-Hall (1964)
[a6]	P.R. Garabedian, "Partial differential equations" , Wiley (1964)