Field operator
A linear weakly-continuous mapping 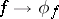 ,
, 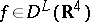 , of the space
, of the space 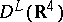 of basic functions
of basic functions 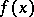 ,
, 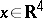 , that take values in a finite-dimensional vector space
, that take values in a finite-dimensional vector space 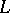 , to the set of operators (generally speaking, unbounded) defined on a dense linear manifold
, to the set of operators (generally speaking, unbounded) defined on a dense linear manifold  of some Hilbert space
of some Hilbert space 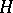 . Here it is assumed that both in
. Here it is assumed that both in 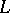 and in
and in  certain representations
certain representations 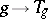 (in
(in  ) and
) and 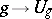 (in
(in  ),
),  , of the inhomogeneous Lorentz group
, of the inhomogeneous Lorentz group 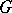 act in such a way that the equation
act in such a way that the equation
 | (*) |
holds, where
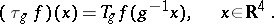 |
Depending on the representation (scalar, vector, spinor, etc.) in  , the field
, the field 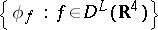 is called, respectively, scalar, vector or spinor. A family of field operators
is called, respectively, scalar, vector or spinor. A family of field operators  together with representations
together with representations 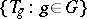 and
and 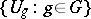 for which condition (*) holds together with several general conditions (see [1]) is called a quantum (or quantized) field.
for which condition (*) holds together with several general conditions (see [1]) is called a quantum (or quantized) field.
Aside from some models referring to the two-dimensional or three-dimensional world (see [2], [4]), one has constructed only (1983) simple examples of so-called free quantum fields [3].
References
| [1] | R. Jost, "The general theory of quantized fields" , Amer. Math. Soc. (1965) |
| [2] | B. Simon, "The 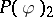 -Euclidean (quantum) field theory" , Princeton Univ. Press (1974) -Euclidean (quantum) field theory" , Princeton Univ. Press (1974) |
| [3] | N.N. Bogolyubov, D.V. Shirkov, "Introduction to the theory of quantized fields" , Interscience (1959) (Translated from Russian) |
| [4] | , Euclidean quantum field theory. The Markov approach , Moscow (1978) (In Russian; translated from English) |
Comments
References
| [a1] | P.J.M. Bongaarts, "The mathematical structure of free quantum fields. Gaussian fields" E.A. de Kerf (ed.) H.G.J. Pijls (ed.) , Proc. Seminar. Mathematical structures in field theory , CWI, Amsterdam (1987) pp. 1–50 |
Field operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Field_operator&oldid=46919