Feynman measure
From Encyclopedia of Mathematics
A complex pre-measure defined on cylindrical sets in the space of functions 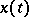 ,
, 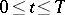 ,
,  , with values in
, with values in 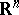 ,
,  by the formula
by the formula
 | (1) |
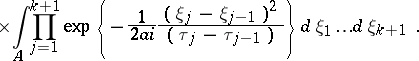 |
Here 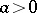 is a parameter,
is a parameter, 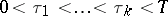 , and
, and
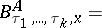 |
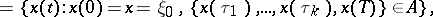 |
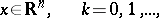 |
where 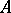 is some Borel subset in
is some Borel subset in 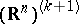 . Sometimes one also considers the so-called conditional Feynman measure
. Sometimes one also considers the so-called conditional Feynman measure 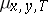 obtained from the measure (1) by restricting it to the set of trajectories with "end" at the point
obtained from the measure (1) by restricting it to the set of trajectories with "end" at the point 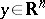 :
: 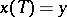 . The measure
. The measure 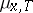 , and also
, and also 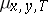 , was introduced by R.P. Feynman in connection with representing the semi-group
, was introduced by R.P. Feynman in connection with representing the semi-group 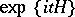 , where
, where 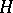 is a Sturm–Liouville operator, in the form of a path integral — a Feynman integral.
is a Sturm–Liouville operator, in the form of a path integral — a Feynman integral.
References
| [1] | R.P. Feynman, "Space-time approach to non-relativistic quantum mechanics" Rev. Modern Phys. , 20 (1948) pp. 367–387 |
| [2] | Yu.L. Daletskii, "Integration in function spaces" Progress in Mathematics , 4 (1969) pp. 87–132 Itogi Nauk. Mat. Anal. 1966 (1967) pp. 83–124 |
| [3] | S.A. Albeverio, R.J. Høegh-Krohn, "Mathematical theory of Feynman path integrals" , Springer (1976) |
How to Cite This Entry:
Feynman measure. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Feynman_measure&oldid=46916
Feynman measure. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Feynman_measure&oldid=46916
This article was adapted from an original article by R.A. Minlos (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article