Extremal properties of polynomials

Properties of algebraic, trigonometric or generalized polynomials that distinguish them as solutions of some extremal problems.

For example, the Chebyshev polynomials have minimal norm in the space among all algebraic polynomials of degree with leading coefficient (P.L. Chebychev, 1853); therefore they are the solution of the extremal problem

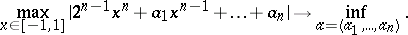In other words, the polynomial differs least from zero in the space among all polynomials of degree with leading coefficient equal to .

Extremal problems in spaces of polynomials are mainly studied in the spaces , . In this context most of the available results are connected with the cases , 2 and 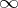(the metric of ). In particular, these metrics are used to find the explicit form of the polynomials that differ least from zero. In the metric of these are the Chebychev polynomials of the second kind, in the metric of one obtains the Legendre polynomials; concerning the metric of 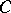see above. The set of classical orthogonal polynomials deviating least from zero in a weighted -space (Laguerre polynomials; Hermite polynomials; Jacobi polynomials; etc.) has also been described.

E.I. Zolotarev (1877) considered the question of determining polynomials of the form 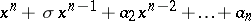(with two fixed leading coefficients) that deviate least from zero in the metric of . He found a one-parameter family of polynomials that solved this problem, and expressed them in terms of elliptic functions.

The Chebychev polynomials are extremal in the problem of an inequality for the derivatives; namely, the exact Markov inequality (where 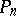is a polynomial of degree )

(*)

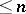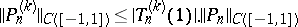holds, with equality for 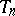. Inequality (*) was proved by A.A. Markov (1889) for , and by V.A. Markov (1892) for all other values of . Concerning a similar inequality for trigonometric polynomials see Bernstein inequality.

Some extremal properties of algebraic and trigonometric polynomials in the uniform metric carry over to Chebychev systems of functions (see [2]). Concerning the theory of extremal problems and extremal properties of polynomials see [6].

References

[1]	P.L. Chebychev, "Oeuvres" , 1–2 , Chelsea, reprint (No date)
[2]	S.N. Bernshtein, "Leçons sur les propriétés extrémales de la meilleure approximation des fonctions analytiques d'une variable réelle" , Chelsea, reprint (1970) (Translated from Russian)
[3]	V.L. Goncharov, "The theory of interpolation and approximation of functions" , Moscow (1954) (In Russian)
[4]	N.I. [N.I. Akhiezer] Achiezer, "Theory of approximation" , F. Ungar (1956) (Translated from Russian)
[5]	E.V. Voronovskaya, "The functional method and its applications" , Amer. Math. Soc. (1970) (Translated from Russian)
[6]	V.M. Tikhomirov, "Some problems in approximation theory" , Moscow (1976) (In Russian)

References

[a1]	E.W. Cheney, "Introduction to approximation theory" , Chelsea, reprint (1982)
[a2]	I.P. Natanson, "Constructive function theory" , 1–3 , F. Ungar (1964–1965) (Translated from Russian)
[a3]	T.J. Rivlin, "The Chebyshev polynomials" , Wiley (1974)
[a4]	H.S. Shapiro, "Topics in approximation theory" , Springer (1971)
[a5]	G.G. Lorentz, "Approximation of functions" , Holt, Rinehart & Winston (1966)