Extension of an associative algebra
over a commutative ring 
A homomorphism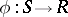 
of a 
-algebra 
onto 
. If 
is an algebra with zero multiplication, then the extension is called singular. In this case, 
is an 
-module in a natural way. The set of all extensions of 
with kernel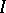 
admits an equivalence relation (the same as for groups, modules, etc.), and the set of equivalence classes of extensions is denoted by 
. If the algebra 
is 
-projective, then the algebra 
splits into a direct sum of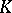 
-modules, 
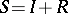, and the elements of 
can be written as pairs 
, 
, 
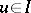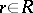, with multiplication given by
 |
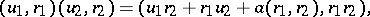where 
. The associativity of multiplication imposes restrictions on 
that make it into a cocycle. Mapping an extension to its cocycle defines an isomorphism of 
-modules between 
and the second cohomology group 
of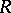 
with coefficients in 
.
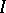In a completely different context, any algebra containing 
is also called an extension of 
. Such extensions are often connected with a specific construction (polynomials over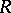 
, localization of 
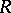, ring of partial fractions of the algebra 
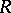, etc.). See also Extension of a field.
References
| [1] | S. MacLane, "Homology" , Springer (1963) |
| [2] | G. Hochschild, "On the cohomology groups of an associative algebra" Ann. of Math. , 46 (1945) pp. 58–67 |
Comments
The cohomology group 
is also known as the Hochschild cohomology (group) of 
with values in 
.
Extension of an associative algebra. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Extension_of_an_associative_algebra&oldid=46882