Exhaustion of a domain
approximating sequence of domains
For a given domain 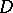 in a topological space
in a topological space  , an exhaustion is a sequence of (in a certain sense regular) domains
, an exhaustion is a sequence of (in a certain sense regular) domains  such that
such that  and
and 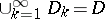 . For any domain
. For any domain 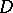 in a complex space
in a complex space 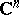 there exists an exhaustion by domains
there exists an exhaustion by domains 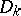 that are, e.g., bounded by piecewise-smooth curves (in
that are, e.g., bounded by piecewise-smooth curves (in 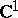 ) or by piecewise-smooth surfaces (in
) or by piecewise-smooth surfaces (in 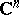 ,
, 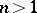 ). For any Riemann surface
). For any Riemann surface 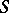 there is a polyhedral exhaustion
there is a polyhedral exhaustion  , consisting of polyhedral domains
, consisting of polyhedral domains 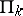 that are, each individually, connected unions of a finite number of triangles in a triangulation of
that are, each individually, connected unions of a finite number of triangles in a triangulation of  ; moreover,
; moreover, 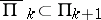 ,
,  , and the boundary of each of the domains making up the open set
, and the boundary of each of the domains making up the open set  is, for sufficiently large
is, for sufficiently large 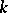 , just one of the boundary contours of
, just one of the boundary contours of 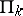 .
.
References
| [1] | S. Stoilov, "The theory of functions of a complex variable" , 2 , Moscow (1962) pp. Chapt. 5 (In Russian; translated from Rumanian) |
Comments
The fact that any pseudo-convex domain (cf. Pseudo-convex and pseudo-concave) can be exhausted by smooth, strictly pseudo-convex domains is of fundamental importance in higher-dimensional complex analysis, cf. [a2].
References
| [a1] | G. Springer, "Introduction to Riemann surfaces" , Addison-Wesley (1957) pp. Chapt.10 |
| [a2] | L.V. Ahlfors, L. Sario, "Riemann surfaces" , Princeton Univ. Press (1960) pp. Chapt. 1 |
| [a3] | S.G. Krantz, "Function theory of several complex variables" , Wiley (1982) pp. Sect. 7.1 |
Exhaustion of a domain. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Exhaustion_of_a_domain&oldid=46873