Ergodic set

in the phase space 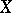(a metrizable compactum) of a topological dynamical system 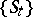(a flow (continuous-time dynamical system) or a cascade) corresponding to a normalized ergodic invariant measure

A set of points 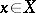such that:

a) for every continuous function 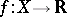the "time average"

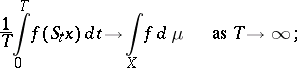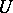b) for every neighbourhood of .

A point for which the limit of the time average in a) exists for every continuous 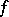is called quasi-regular. For such a point this limit has the form 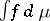, where is some normalized invariant measure, depending on and not necessarily ergodic. If b) holds for this 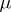, then the point is called a density point, if this 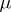is ergodic, then the point is called transitive; when both conditions hold, the point is called regular. The set of non-regular points has measure zero relative to any normalized invariant measure. The partition of the set of regular points into ergodic sets corresponding to different is the strongest realization of the idea of decomposing a dynamical system into ergodic components (which is valid under stronger restrictions on the system).

Ergodic sets were introduced by N.N. Bogolyubov and N.M. Krylov [1]. For other accounts, discussions of various generalizations and related questions see the references to Invariant measure 1) and Metric transitivity.

References

[1]	N. Krylov, N. [N.N. Bogolyubov] Bogoliouboff, "La théorie générale de la mesure dans son application à l'étude des systèmes dynamiques de la mécanique non-linéare" Ann. of Math. Ser. (2) , 38 (1937) pp. 65–113

Comments

A good account of ergodic sets is given in [a1]. Closely related is the notion of a generic point (with respect to a normalized invariant measure 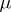): A quasi-regular point such that for every continuous 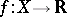the limit in a) is , where 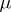is the given measure.

References

[a1]	J.C. Oxtoby, "Ergodic sets" Bull. Amer. Math. Soc. , 58 (1952) pp. 116–136