Equivariant cohomology
A cohomology that takes the action of some group into account. More precisely, an equivariant cohomology in the category of  -spaces (that is, topological spaces on which the continuous action of a group
-spaces (that is, topological spaces on which the continuous action of a group  is defined) and equivariant mappings is a sequence of contravariant functors
is defined) and equivariant mappings is a sequence of contravariant functors  (taking values in the category of Abelian groups) and natural transformations
(taking values in the category of Abelian groups) and natural transformations
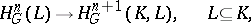 |
with the following properties: a) equivariantly-homotopic mappings of pairs induce identity homomorphisms of the groups  ; b) an inclusion of the form
; b) an inclusion of the form
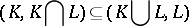 |
induces an isomorphism
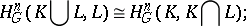 |
and c) for every pair 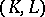 the following cohomology sequence is exact:
the following cohomology sequence is exact:
 |
Let  be a universal
be a universal  -fibration and let
-fibration and let  be the space associated with the universal fibre space
be the space associated with the universal fibre space 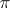 with fibre
with fibre  (that is, the quotient space
(that is, the quotient space  under the action of
under the action of  given by
given by 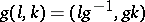 ). Then the functors
). Then the functors  yield an equivariant cohomology theory; here
yield an equivariant cohomology theory; here  is an arbitrary cohomology theory.
is an arbitrary cohomology theory.
For any fixed group  the collection of groups
the collection of groups  together with all possible homomorphisms induced by inclusions
together with all possible homomorphisms induced by inclusions  of subgroups of
of subgroups of  is usually called the system of coefficients for the
is usually called the system of coefficients for the  -theory. In some cases the functors
-theory. In some cases the functors  are uniquely defined by their systems of coefficients (for example, when
are uniquely defined by their systems of coefficients (for example, when  is finite and
is finite and  for
for  ).
).
References
| [1] | G.E. Bredon, "Equivariant cohomology theories" , Springer (1967) |
| [2] | W.Y. Hsiang, "Cohomology theory of topological transformation groups" , Springer (1975) |
Comments
The principal use of equivariant cohomology is in equivariant obstruction theory and in such problems of equivariant (stable) homotopy theory as the solution of the Segal conjecture by G. Carlsson [a1] (see also [a2] and Homotopy).
Quite generally, in many parts of mathematics it is useful to consider also family and equivariant versions of various constructions and results. The various family (relative) and equivariant versions of parts of mathematics are then often also important tools in the non-equivariant and non-family settings. An example of this is the use of equivariant  -theory in proofs of the Atiyah–Singer index and fixed-points theorems, cf., e.g., [a3].
-theory in proofs of the Atiyah–Singer index and fixed-points theorems, cf., e.g., [a3].
Thus, many theories, e.g. cohomology theories, have equivariant versions, e.g. equivariant (stable) homotopy theory [a1], equivariant  -theory [a3], [a4], equivariant cobordism [a5], [a7]. And there are equivariant versions of many theorems and constructions such as equivariant surgery [a1], [a7], equivariant smoothing [a6] and equivariant transversality [a7].
-theory [a3], [a4], equivariant cobordism [a5], [a7]. And there are equivariant versions of many theorems and constructions such as equivariant surgery [a1], [a7], equivariant smoothing [a6] and equivariant transversality [a7].
References
| [a1] | G. Carlsson, "Equivariant stable homotopy and Segal's Burnside ring conjecture" Ann. of Math. , 120 (1984) pp. 189–224 |
| [a2] | L.G. Lewis, J.P. May, M. Steinberger, "Equivariant stable homotopy theory" , Lect. notes in math. , 1213 , Springer (1986) (With contributions by J.E. McClure) |
| [a3] | T. Petrie, J.D. Randall, "Transformation groups on manifolds" , M. Dekker (1984) |
| [a4] | T. tom Dieck, "Transformation groups and representation theory" , Springer (1979) |
| [a5] | T. Petrie, "Pseudoequivalences of  -manifolds" R.J. Milgram (ed.) , Algebraic and geometric topology , 33.1 , Amer. Math. Soc. (1978) pp. 169–210 -manifolds" R.J. Milgram (ed.) , Algebraic and geometric topology , 33.1 , Amer. Math. Soc. (1978) pp. 169–210 |
| [a6] | R. Lashof, M. Rothenberg, " -smoothing theory" R.J. Milgram (ed.) , Algebraic and geometric topology , 33.1 , Amer. Math. Soc. (1978) pp. 211–266 -smoothing theory" R.J. Milgram (ed.) , Algebraic and geometric topology , 33.1 , Amer. Math. Soc. (1978) pp. 211–266 |
| [a7] | W. Browder, F. Quinn, "A surgery theory for  -manifolds and stratified sets" , Manifolds (Tokyo) , Univ. Tokyo Press (1973) pp. 27–36 -manifolds and stratified sets" , Manifolds (Tokyo) , Univ. Tokyo Press (1973) pp. 27–36 |
Equivariant cohomology. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Equivariant_cohomology&oldid=46845