Equivalent representations
Two representations 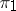 and
and 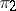 of a group (algebra, ring, semi-group)
of a group (algebra, ring, semi-group)  in vector spaces
in vector spaces 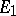 and
and 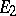 , respectively, for which there is an intertwining operator which is a vector space isomorphism from
, respectively, for which there is an intertwining operator which is a vector space isomorphism from 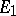 to
to 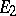 (sometimes such representations are called algebraically equivalent); if
(sometimes such representations are called algebraically equivalent); if 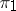 and
and  are representations in topological vector spaces
are representations in topological vector spaces 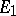 and
and  , then
, then 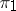 and
and 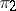 are called topologically equivalent if there is an intertwining operator for
are called topologically equivalent if there is an intertwining operator for 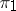 and
and 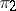 which is a topological vector space isomorphism from
which is a topological vector space isomorphism from 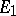 to
to 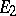 . The term "equivalent representations" is also used to define some other equivalence relations: For example, two representations are called weakly equivalent if there is a closed operator with a dense domain of definition and a dense range that intertwines these representations. Two representations of a Lie group in Banach spaces are called infinitesimally equivalent if the induced representations of the universal enveloping algebra on their spaces of analytic vectors are algebraically equivalent. Two representations of an algebra are sometimes called equivalent, or isomorphic, if their kernels coincide; two representations of a topological group are called equivalent if the induced representations of some group algebra of this group are isomorphic.
. The term "equivalent representations" is also used to define some other equivalence relations: For example, two representations are called weakly equivalent if there is a closed operator with a dense domain of definition and a dense range that intertwines these representations. Two representations of a Lie group in Banach spaces are called infinitesimally equivalent if the induced representations of the universal enveloping algebra on their spaces of analytic vectors are algebraically equivalent. Two representations of an algebra are sometimes called equivalent, or isomorphic, if their kernels coincide; two representations of a topological group are called equivalent if the induced representations of some group algebra of this group are isomorphic.
Equivalent representations. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Equivalent_representations&oldid=46843