EHP spectral sequence

An inductive procedure to calculate the homotopy groups of spheres (cf. Spheres, homotopy groups of the). It has the attractive feature that the input for the calculation is always a result of an earlier calculation once one inputs . It has been used to calculate for . The last of these calculations are contained in [a3] and complete references can be found there. It is constructed by splicing together fibrations discovered by I.M. James [a2] at the prime number and at odd prime numbers by H. Toda [a4].

In this paragraph the case of the prime number is discussed, and all spaces are -local and all groups should be considered as completed at . James showed that there is a -local fibration . This gives rise to long exact sequences (cf. Exact sequence)

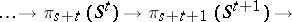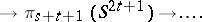The first mapping is usually called , the second and the connecting homomorphism . The EHP spectral sequence is obtained by defining the filtration of by

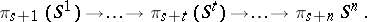This gives rise to a spectral sequence with 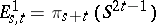and converging to . There are several important features of this spectral sequence. First, note that 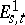is itself a result of another calculation using this spectral sequence for smaller values of . The value of is called the stem and the filtration parameter gives the sphere of origin of a class. The name a homotopy class receives from this spectral sequence is called the Hopf invariant of the class. Letting go to infinity gives a spectral sequence which calculates the stable homotopy groups (cf. Stable homotopy group). The 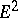term does not have a special name, but there is the following result. 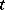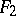For all , , and odd , is an vector space [a1]. The differential is .

For odd prime numbers, the situation is slightly more complicated. In this paragraph all spaces are -local for a fixed odd prime number and all groups are completed at 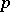. First there is the result of James, . This reduces the calculation for even-dimensional spheres to that of odd-dimensional spheres. In order to get Toda's version of the EHP sequence, one introduces a modified even sphere. Let 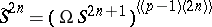, the -skeleton of . Then Toda showed that the following are -local fibrations:

As in the prime case, one can fit the long exact sequences in homotopy together to get a spectral sequence. It is associated to the filtration

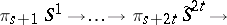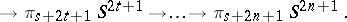As before, one can use Toda's sequences to identify the -term: and . This spectral sequence converges to the 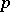-local homotopy of . As before, the input is the result of an earlier calculation. There is also the classical result that 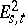is an vector space. The parameter refers to the stem and the parameter refers to the sphere of origin. Here, this refers to the odd sphere or the modified even sphere of origin. The name a class gets in this spectral sequence is also called the Hopf invariant.

References

[a1]	M. Barratt, F. R. Cohen, B. Gray, M. Mahowald, W. Richter, "Two results on the -local EHP spectral sequence" Proc. Amer. Math. Soc. , 123 (1995) pp. 1257–1261
[a2]	I.M. James, "On the suspension sequence" Ann. of Math. , 65 (1957) pp. 74–107
[a3]	N. Oda, "On the -components of the unstable homotopy groups of spheres, I–II" Proc. Japan Acad. Ser. A Math. Sci. , 53 (1977) pp. 202–218
[a4]	H. Toda, "Composition methods in homotopy groups of spheres" , Ann. Math. Studies , 49 , Princeton Univ. Press (1962)