Disc of convergence

of a power series

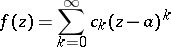The disc 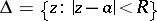, , 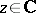in which the series

is absolutely convergent, while outside the disc (for 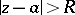) it is divergent. In other words, the disc of convergence 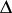is the interior of the set of points of convergence of the series . Its radius 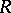is called the radius of convergence of the series. The disc of convergence may shrink to the point when , and it may be the entire open plane, when 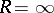. The radius of convergence 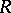is equal to the distance of the centre to the set of singular points of 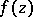(for the determination of 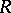in terms of the coefficients 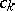of the series see Cauchy–Hadamard theorem). Any disc 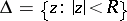, , 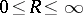in the -plane is the disc of convergence of some power series.

For a power series

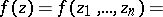(2)

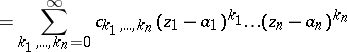in several complex variables , , 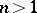a polydisc of convergence of the series (2) is defined to be any polydisc

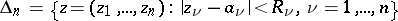at all points of which the series (2) is absolutely convergent, while in any polydisc

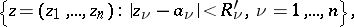where 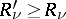and at least one of the latter inequalities is strict, there is at least one point at which the series is divergent. The radii , , 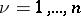, of the polydisc of convergence are called the associated radii of convergence of the series (2). They are in a well-defined relationship with the coefficients of the series, so that any polydisc with centre and with radii satisfying this relationship is the polydisc of convergence of a series (2) (cf. Cauchy–Hadamard theorem). Any polydisc 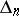, , 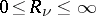, 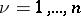in the complex space 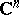is the polydisc of convergence for some power series in complex variables. When the interior of the set of points of absolute convergence of a series (2) is more complicated — it is a logarithmically convex complete Reinhardt domain with centre in 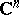(cf. Reinhardt domain).

References

[1]	A.I. Markushevich, "Theory of functions of a complex variable" , 1 , Chelsea (1977) (Translated from Russian)
[2]	B.V. Shabat, "Introduction of complex analysis" , 1 , Moscow (1976) (In Russian)

References

[a1]	L. Hörmander, "An introduction to complex analysis in several variables" , North-Holland (1973) pp. Chapt. 2.4
[a2]	L.V. Ahlfors, "Complex analysis" , McGraw-Hill (1978)
[a3]	W. Rudin, "Real and complex analysis" , McGraw-Hill (1987) pp. 24