Differential equations, infinite-order system of
infinite system of differential equations
An infinite set of differential equations
 | (1) |
containing an infinite set of unknown functions 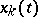 ,
, 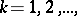 and their derivatives. A solution of such a system is defined as a set of functions
and their derivatives. A solution of such a system is defined as a set of functions 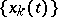 for which all the equations of the system hold identically.
for which all the equations of the system hold identically.
The system (1) is said to be countable, as distinct from the uncountable system
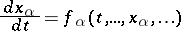 | (2) |
where 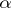 runs through some uncountable set of values. Systems of the type (2) contain an uncountable set of functions
runs through some uncountable set of values. Systems of the type (2) contain an uncountable set of functions 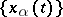 which must be determined as well as their derivatives. Partial differential equations containing an uncountable set of unknown functions in two or more arguments are also studied.
which must be determined as well as their derivatives. Partial differential equations containing an uncountable set of unknown functions in two or more arguments are also studied.
A.N. Tikhonov [1] is the author of the first publication on the theory of systems of differential equations of the type (1). His main result was an existence proof of a solution of (1), under the assumption that its right-hand sides are defined for arbitrary values 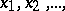
 , are continuous with respect to
, are continuous with respect to  for a given value of
for a given value of 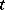 , and are measurable with respect to
, and are measurable with respect to 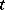 for given
for given  on an interval
on an interval 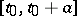 . If, in addition, the generalized Lipschitz conditions
. If, in addition, the generalized Lipschitz conditions
 |
are met, and the series
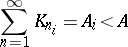 |
converge and are uniformly bounded, then the solution 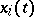 ,
,  of (1) is unique if the given initial conditions are such that the series
of (1) is unique if the given initial conditions are such that the series
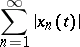 |
converges.
The subsequent development of the theory of countable systems concerned the conditions of boundedness of the solutions [2], analytic dependence on the parameter, Lyapunov stability and other properties of the solutions [2]. Linear and quasi-linear countable systems of differential equations have been particularly thoroughly studied.
Operator methods are especially fruitful in the study of systems of infinite order. For instance, rather than the system (1) one considers the operator equation
 | (3) |
where 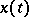 is an infinite-dimensional vector of some Banach space
is an infinite-dimensional vector of some Banach space 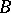 ,
, 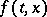 is an infinite-dimensional vector-function with values in this space, and the derivative is considered in the sense of Fréchet. In particular, the following results were obtained [3] for equation (3).
is an infinite-dimensional vector-function with values in this space, and the derivative is considered in the sense of Fréchet. In particular, the following results were obtained [3] for equation (3).
If 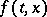 is a bounded operator, it follows from the validity of the local existence theorem that if the Bohl exponent at zero is negative [3], then the solutions with initial values close to zero can be defined on arbitrary large intervals.
is a bounded operator, it follows from the validity of the local existence theorem that if the Bohl exponent at zero is negative [3], then the solutions with initial values close to zero can be defined on arbitrary large intervals.
If
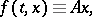 |
where  is a bounded operator given by an infinite-dimensional matrix, then all solutions are bounded for
is a bounded operator given by an infinite-dimensional matrix, then all solutions are bounded for 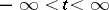 in a Hilbert space if and only if
in a Hilbert space if and only if 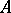 is similar to a skew-Hermitian matrix. In this case, the explicit form of the solution of the Cauchy problem with initial conditions
is similar to a skew-Hermitian matrix. In this case, the explicit form of the solution of the Cauchy problem with initial conditions 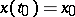 was found in the form
was found in the form
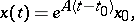 |
where 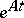 is the operator exponent.
is the operator exponent.
If
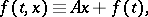 |
where 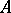 has its previous meaning while
has its previous meaning while 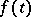 is a continuous
is a continuous 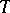 -periodic vector-function, there exists a unique periodic solution when the spectrum
-periodic vector-function, there exists a unique periodic solution when the spectrum 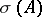 of
of  does not contain the points
does not contain the points 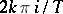 ,
,  of the imaginary axis.
of the imaginary axis.
Conditions for Lyapunov stability at zero were found for the case
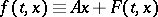 |
in the form of the requirement
 |
for a sufficiently small  , if the spectrum of
, if the spectrum of 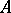 lies in the open left half-space.
lies in the open left half-space.
References
| [1] | A.N. Tikhonov, Mat. Sb. , 41 : 4 (1934) pp. 551–560 |
| [2] | K.G. Valeev, O.A. Zhautykov, "Infinite systems of differential equations" , Alma-Ata (1974) (In Russian) |
| [3] | Yu.L. Daletskii, M.G. Krein, "Stability of solutions of differential equations in Banach space" , Amer. Math. Soc. (1974) (Translated from Russian) |
Comments
Consider a homogeneous differential equation
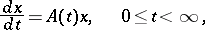 | (a1) |
where 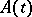 is an operator-valued function,
is an operator-valued function,  an operator on the Banach space
an operator on the Banach space 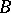 ,
, 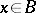 . Let
. Let
 |
be a solution, 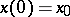 . The (upper) Bohl exponent
. The (upper) Bohl exponent  of this solution is the greatest lower bound of all real numbers
of this solution is the greatest lower bound of all real numbers 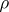 such that there exists an
such that there exists an 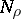 for which
for which
 |
for all 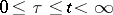 . The lower Bohl exponent
. The lower Bohl exponent 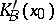 is the least upper bound of the numbers
is the least upper bound of the numbers 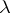 for which there exists an
for which there exists an 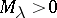 such that
such that
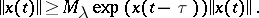 |
If 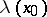 is a Lyapunov exponent of (a1), cf. Lyapunov characteristic exponent, then
is a Lyapunov exponent of (a1), cf. Lyapunov characteristic exponent, then
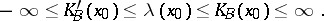 |
The interval  is called the Bohl interval of the solution in question.
is called the Bohl interval of the solution in question.
Now consider again the equation (3) and let 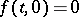 . This equation is said to satisfy property
. This equation is said to satisfy property 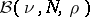 ,
, 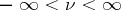 ,
, 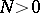 ,
, 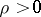 , if every solution of it with
, if every solution of it with 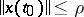 at some time
at some time  , satisfies the estimate
, satisfies the estimate
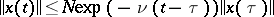 |
for all  for which the solution is defined. Generalizing the definitions above, the (upper) Bohl exponent at zero is the greatest lower bound of the
for which the solution is defined. Generalizing the definitions above, the (upper) Bohl exponent at zero is the greatest lower bound of the 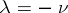 for which there exist
for which there exist 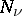 ,
,  such that the equation has property
such that the equation has property 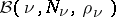 .
.
References
| [a1] | A. Pazy, "Differential equations in Banach spaces" , Springer (To appear) |
Differential equations, infinite-order system of. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Differential_equations,_infinite-order_system_of&oldid=46681