Differentiability of solutions (of differential equations)
A property of solutions of differential equations, viz. that the solutions posses a specific number of continuous derivatives with respect to the independent variable 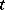 and the parameter
and the parameter 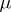 appearing in the equation. In the theory of differential equations the problem is posed as follows: What are the properties which the right-hand side of the equation must have for the solution to have a given number of continuous derivatives with respect to
appearing in the equation. In the theory of differential equations the problem is posed as follows: What are the properties which the right-hand side of the equation must have for the solution to have a given number of continuous derivatives with respect to 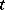 and
and 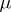 ? This problem has been most thoroughly investigated for ordinary differential equations [1], [4].
? This problem has been most thoroughly investigated for ordinary differential equations [1], [4].
Consider an equation of the type ( may also be a vector):
may also be a vector):
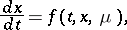 | (1) |
where 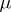 is a parameter (usually also a vector), and let
is a parameter (usually also a vector), and let 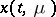 be a solution of (1) defined by the initial condition
be a solution of (1) defined by the initial condition
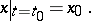 | (2) |
First differentiability of the solution with respect to 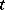 is considered. If
is considered. If  is continuous with respect to
is continuous with respect to 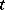 and
and  , the theorem on the existence of a continuous solution of the problem (1)–(2) is applicable in some domain, and then it follows from the identity which is obtained after substitution of
, the theorem on the existence of a continuous solution of the problem (1)–(2) is applicable in some domain, and then it follows from the identity which is obtained after substitution of 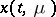 in (1) that the continuous derivative
in (1) that the continuous derivative 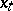 also exists. The presence of
also exists. The presence of  continuous derivatives of
continuous derivatives of 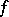 with respect to
with respect to 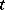 and
and  means that there exist
means that there exist 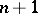 continuous derivatives of the solution with respect to
continuous derivatives of the solution with respect to 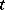 ;
; 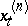 may be found (expressed in terms of
may be found (expressed in terms of  ) by successive differentiation of the identity obtained by substituting
) by successive differentiation of the identity obtained by substituting  in (1).
in (1).
In several problems, e.g. in constructing the asymptotics of the solution in the parameter 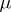 , it is necessary to study derivatives of
, it is necessary to study derivatives of 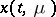 with respect to
with respect to 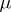 . In order to be specific, the existence of derivatives with respect to
. In order to be specific, the existence of derivatives with respect to  for
for 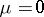 will be considered. If
will be considered. If 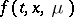 is continuous and has continuous partial derivatives with respect to
is continuous and has continuous partial derivatives with respect to  and
and 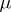 in some domain,
in some domain, 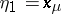 exists and is defined from the so-called variational equation (equation in variations, which is linear in
exists and is defined from the so-called variational equation (equation in variations, which is linear in  ), obtained from (1) by differentiating both parts with respect to
), obtained from (1) by differentiating both parts with respect to  and putting
and putting 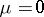 :
:
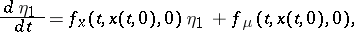 | (3) |
and with the aid of the initial condition
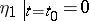 | (4) |
if 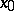 is independent of
is independent of 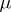 ; if, however,
; if, however,  , then
, then 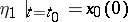 .
.
The derivative 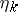 of
of  with respect to
with respect to 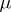 of order
of order 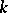 (under the condition that
(under the condition that 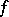 has continuous partial derivatives up to order
has continuous partial derivatives up to order 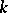 ) is defined by the variational equation of the
) is defined by the variational equation of the 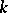 -th order, which differs from (3) only in its inhomogeneity, and it depends on
-th order, which differs from (3) only in its inhomogeneity, and it depends on 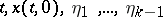 . In the presence of
. In the presence of 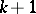 continuous derivatives of
continuous derivatives of 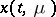 with respect to
with respect to 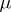 , Taylor's formula may be used as the asymptotic formula for
, Taylor's formula may be used as the asymptotic formula for 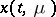 with respect to
with respect to 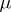 :
:
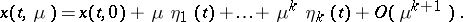 | (5) |
This is very important, since 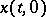 and
and 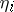 can then be found from equations simpler than (1).
can then be found from equations simpler than (1).
If the right-hand side depends analytically on its arguments, the solution is an analytic function of the parameter 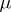 (see, for example, [2]).
(see, for example, [2]).
The problem of differentiability of solutions with respect to  is still meaningful in several cases when the right-hand side does not depend regularly on
is still meaningful in several cases when the right-hand side does not depend regularly on 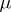 . In one such case
. In one such case 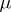 appears as the coefficient in front of the derivative:
appears as the coefficient in front of the derivative:
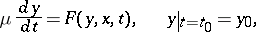 | (6) |
 |
If (6) is rewritten in the form (1), i.e. is solved with respect to the derivatives, a pole-type singularity appears on the right-hand side as  . It is found that, in the presence of
. It is found that, in the presence of  continuous derivatives of the right-hand sides and under certain special conditions (the so-called stability conditions), expansion (5) is valid, where
continuous derivatives of the right-hand sides and under certain special conditions (the so-called stability conditions), expansion (5) is valid, where 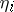 are the limit values of the derivatives with respect to
are the limit values of the derivatives with respect to 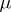 of the solution of (6) as
of the solution of (6) as 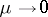 , which are defined by the variational equation constructed according to the same rule: (6) is differentiated with respect to
, which are defined by the variational equation constructed according to the same rule: (6) is differentiated with respect to  and
and 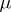 is set equal to zero. However, as distinct from the regular case, the system of variational equations will be of a lower order than (6), and the initial values for
is set equal to zero. However, as distinct from the regular case, the system of variational equations will be of a lower order than (6), and the initial values for 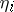 will no longer be zero — but will be equal to (usually non-zero) constants, obtained by a definite rule [3].
will no longer be zero — but will be equal to (usually non-zero) constants, obtained by a definite rule [3].
References
| [1] | I.G. Petrovskii, "Ordinary differential equations" , Prentice-Hall (1966) (Translated from Russian) |
| [2] | A.N. Tikhonov, "On the dependence of solutions of differential equations on a small parameter" Mat. Sb. , 22 : 2 (1948) pp. 193–204 (In Russian) |
| [3] | A.B. Vasil'eva, V.F. Butuzov, "Asymptotic expansions of solutions of singularly perturbed equations" , Moscow (1973) (In Russian) |
| [4] | A.N. Tikhonov, A.B. Vasil'eva, A.G. Sveshnikov, "Differential equations" , Springer (1985) (Translated from Russian) |
Comments
References
| [a1] | E.A. Coddington, N. Levinson, "Theory of ordinary differential equations" , McGraw-Hill (1955) pp. Chapts. 13–17 |
| [a2] | D.R. Smith, "Singular perturbation theory" , Cambridge Univ. Press (1985) |
Differentiability of solutions (of differential equations). Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Differentiability_of_solutions_(of_differential_equations)&oldid=46658