Degenerate game

separable game, polynomial-like game

A non-cooperative game of persons in which the pay-off function of each player 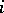is degenerate, i.e. has the form

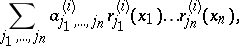where 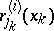, , 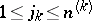are functions defined on the set of pure strategies 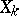of player 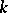, . In the case of two-person zero-sum degenerate games on the unit square the pay-off function 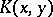of player I is

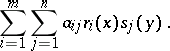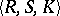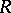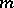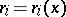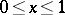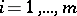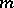Such a game is reduced to a finite two-person zero-sum convex game , where is the convex set spanned by the -dimensional curve , , , in -dimensional space, while 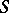is the convex set spanned by the curve 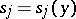, , , 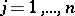in -dimensional space; the pay-off function 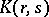has the form

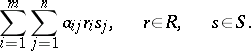In particular, if and 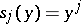, the degenerate game is called a polynomial game. In any two-person zero-sum degenerate game on the unit square player I has an optimal mixed strategy whose support consists of at most 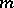points and if the game is polynomial — of at most points (in computing the number of points the weight assigned to a terminal point is ). In a similar manner, player II has an optimal mixed strategy whose support consists of at most points, and in the case of a polynomial game — of at most 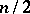points.

References

[1]	M. Dresher, S. Karlin, L.S. Shapley, "Polynomial games" , Contributions to the theory of games I , Ann. Math. Studies , 24 , Princeton Univ. Press (1950) pp. 161–180
[2]	D. Gale, O. Gross, "A note on polynomial and separable games" Pacific J. Math. , 8 : 4 (1958) pp. 735–741