Cylinder coordinates
From Encyclopedia of Mathematics
cylindrical coordinates
Numbers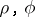 
and 
connected with the Cartesian coordinates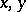 
and 
by the formulas:
 |
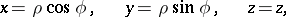where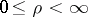 
, 
, 
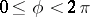. The coordinate surfaces (see Fig.) are: circular cylinders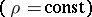 
, half-planes 
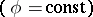and planes 
.
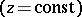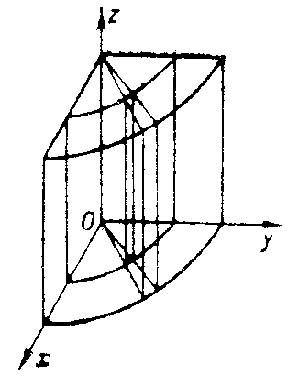
Figure: c027600a
The system of cylinder coordinates is orthogonal.
The Lamé coefficients are:
 |
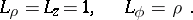The area element of a surface is:
 |
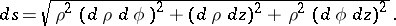The volume element is:
 |
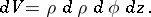The differentiation operations of vector analysis are given by:
 |
 |
 |
 |
 |
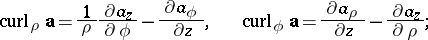Generalized cylinder coordinates are numbers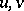 
and 
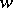connected with Cartesian coordinates 
and 
by the formulas
 |
where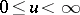 
, 
, 
, 
, 
, 
, 
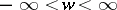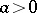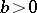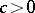. The coordinate surfaces are: elliptic cylinders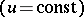 
, half-planes 
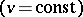and planes 
.
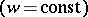Comments
References
| [a1] | U.G. Chambers, "A course in vector analysis" , Chapman & Hall (1969) |
How to Cite This Entry:
Cylinder coordinates. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Cylinder_coordinates&oldid=46573
Cylinder coordinates. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Cylinder_coordinates&oldid=46573
This article was adapted from an original article by D.D. Sokolov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article