Crossed homomorphism
of a group 
into a group 
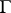with group of operators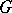 
A mapping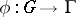 
satisfying the condition 
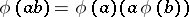. If 
acts trivially on 
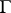, then crossed homomorphisms are just ordinary homomorphisms. Crossed homomorphisms are also called 
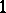-cocycles of 
with values in 
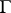(see Non-Abelian cohomology). Every element 
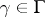defines a crossed homomorphism 
(
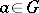), called a principal crossed homomorphism, or cocycle cohomologous to 
. A mapping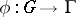 
is a crossed homomorphism if and only if the mapping 
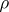of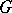 
into the holomorph of 
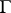(cf. Holomorph of a group) given by 
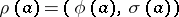, where 
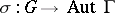is the homomorphism defining the 
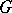action on 
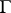, is a homomorphism. For example, if 
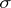is a linear representation of 
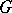in a vector space 
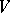, then any crossed homomorphism 
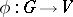defines a representation 
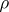of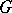 
by affine transformations of 
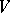. The set 
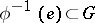is called the kernel of the crossed homomorphism 
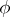; it is always a subgroup of 
.
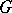Comments
References
| [a1] | S. MacLane, "Homology" , Springer (1963) |
| [a2] | S. Lang, "Rapport sur la cohomologie des groupes" , Benjamin (1966) |
Crossed homomorphism. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Crossed_homomorphism&oldid=46557