Credibility theory

A branch of actuarial mathematics which explores principles for individual experience rating of insurance contracts by certain linear formulas. It emanated in 1918 from a work of A.W. Whitney [a1], who proposed that the rate of premium currently charged for an individual insurance contract be a weighted average of the form

where is the observed mean claim amount per unit of risk exposed for the individual and is the corresponding overall mean in the insurance portfolio (cf. also Risk theory). The weight , which is a number between and , was soon named credibility (or credibility factor), since it measures the "amount of credence attached to the individual experience" , and was called the credibility premium (or credibility premium rate).

Attempts to establish a theoretical basis for rating by credibility formulas bifurcated into two branches. First, the so-called limited fluctuation credibility theory took the point of view that the underlying true individual premium, , say, is a fixed, unknown parameter to be estimated. It focused in particular on developing criteria for "full credibility" , . This branch of the theory faded around 1960. For an overview see [a2].

The other branch of credibility theory stuck to Whitney's original view of as a random variable and developed into what, in the wider perspective of contemporary (1996) statistical decision theory, is linear Bayes and linear empirical Bayes estimation applied to insurance problems (cf. also Bayesian approach). The basic paradigm was already set up in Robbins' empirical Bayes theory and Kalman's linear filtering theory when, at the end of the 1960s, H. Bühlmann in two seminal papers [a6], [a7] formulated the credibility problem as that of minimizing the mean-squared error 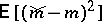over all inhomogeneous linear functions , with and constants. Under the assumption of conditional unbiasedness of the individual claims ratio, 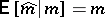, the solution is the credibility premium 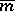above, with and . The mean-squared error criterion applied to linear formulas amounts to minimizing a positive-definite quadratic form in the coefficients. This simple optimization problem could readily be carried over to more complex models and wider classes of linear premium formulas, and a rapid development now followed in this branch of the theory, which received the name greatest accuracy credibility theory. Its evolutionary history paralleled, and partly preceded, that of its statistics counterpart, notably as regards Hilbert space methods [a3], hierarchical and dynamical models, and schemes for recursive computation.

A survey of the history of credibility theory up to 1979 is given in [a4]. An up-to-date introduction to the field is [a5].

References

[a1]	A.W. Whitney, "The theory of experience rating" Proc. Casualty Actuarial Soc. , 4 (1918) pp. 274–292
[a2]	L.H. Longley-Cook, "An introduction to credibility theory" Proc. Casualty Actuarial Soc. , 49 (1962) pp. 194–221
[a3]	F. de Vylder, "Geometrical credibility" Scand. Actuarial J. (1976) pp. 121–149
[a4]	R. Norberg, "The credibility approach to experience rating" Scand. Actuarial J. (1979) pp. 181–221
[a5]	B. Sundt, "An introduction to non-life insurance mathematics" , VVW e. V. Karlsruhe (1993)
[a6]	H. Bühlmann, "Experience rating and credibility" ASTIN Bull. , 4 (1967) pp. 199–207
[a7]	H. Bühlmann, "Experience rating and credibility" ASTIN Bull. , 5 (1969) pp. 157–165