Cramer rule

If the determinant 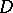of a square system of linear equations

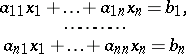does not vanish, then the system has a unique solution. This solution is given by the formulas

(*)

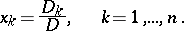Here 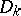is the determinant obtained from when the 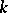-th column is replaced by the column of the free terms 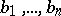. Formulas (*) are known as Cramer's formulas. They have been found by G. Cramer (see [1]).

References

[1]	G. Cramer, "Introduction à l'analyse des lignes courbes" , Geneva (1750) pp. 657
[2]	A.G. Kurosh, "Higher algebra" , MIR (1972) (Translated from Russian)

References

[a1]	T.M. Apostol, "Calculus" , 2 , Wiley (1969) pp. 93