Conformal mapping, boundary properties of a

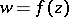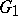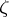Properties of functions mapping one domain in the complex plane conformally onto another that show up near the boundary of the mapped domain and on the boundary itself. Among such properties are: the possibility of continuously extending a function mapping a given domain conformally onto a domain to some point of the boundary of 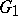or to the entire boundary of this domain; the nature of the discontinuity in the case where such an extension is not possible; conformality of the extended mapping at the boundary points ; the differentiability or smoothness properties of the extended function on 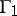and on the closed domain 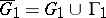; or the membership of the derivative of the mapping function to various classes of analytic functions in 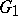, etc. These properties are studied in their dependence on the properties of the boundaries of 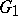and 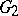. From among the most general boundary properties of conformal mappings one can distinguish: For any simply-connected domains 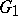and 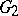and any univalent conformal mapping 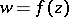of onto 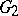, this mapping sets up a one-to-one correspondence between the prime ends (cf. Limit elements) of these domains in the sense that the class of all equivalent paths lying in 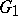and defining some prime end 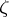of 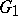is taken by this mapping into the class of all equivalent paths lying in 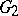and defining some prime end 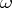of 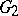(the inverse mapping 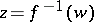, , 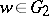takes the class of equivalent paths defining 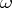into the class of equivalent paths defining ). Furthermore, 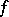determines, in a special topology, a homeomorphism of the domain with its prime ends adjoined (regarded together with the points 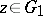as points of a topological space) onto the domain with prime ends adjoined. One usually considers the case when one of the domains , 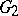is the unit disc 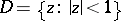(more rarely, the half-plane or a sector); the general case reduces to this particular case.

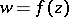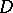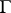Let be a univalent conformal mapping of the disc with boundary onto a bounded domain with boundary , let 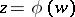be its inverse: 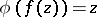for . Then one has the following results.

1) In order that be continuously extendable to a point 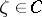it is necessary and sufficient that the prime end of 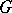corresponding to 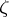under this mapping be a prime end of the first kind (that is, it consists of a single point). In order that be continuously extendable to a point 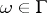it is necessary and sufficient that 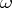be part of just one prime end (more precisely, be part of just one support of a prime end of ). If 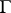is a closed Jordan curve, then is continuously extendable onto 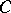, and onto 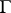, so that the extended functions realize a one-to-one bicontinuous mapping (a homeomorphism) of the closed domains 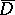, 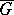onto each other.

In what follows 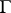denotes a Jordan curve and it is supposed that the functions 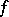and 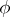are extended onto and , respectively.

2) If 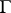is a closed rectifiable Jordan curve, then the boundary functions 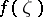, , 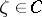and 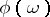, , are absolutely continuous. Thus, , , 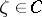and , , take boundary sets of measure zero to boundary sets of measure zero. The function 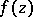has a finite non-zero derivative relative to the closed disc 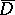at almost-every point , while has a finite non-zero derivative at almost-every point 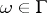. Consequently, these mappings are conformal (that is, have the property of constant dilation and preservation of angles) at almost-every boundary point of their respective domains. The function 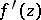belongs to the Hardy class .

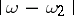3) Let be a closed rectifiable Jordan curve with the following property: For any distinct points , the ratio of the length of the smaller of the arcs into which these points divide to the distance between these points is bounded from above by some quantity 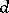that does not depend on or 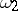. Then 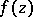satisfies the Hölder condition of order 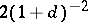on .

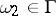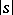4) Let be a smooth closed Jordan curve. A point is fixed and for an arc of length is laid-off along 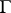in the positive (when ) or negative (when 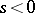) direction of traversal of 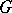. Let 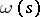be the end of the arc laid-off, and let 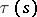be the angle between the positive direction of the real axis and the positive direction of the tangent at 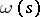(the value of is chosen so that the function 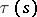is continuous). 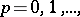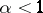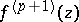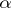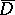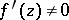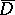If there exists for some a derivative satisfying a Hölder condition of some positive order , then the function is continuous and satisfies the Hölder condition of the same order on the closed disc , moreover on and 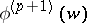is continuous and satisfies the Hölder condition of order 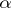on 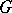with, moreover, 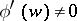on .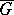

References

[1]	A.I. Markushevich, "Theory of functions of a complex variable" , 3 , Chelsea (1977) (Translated from Russian)
[2]	E.F. Collingwood, A.J. Lohwater, "The theory of cluster sets" , Cambridge Univ. Press (1966) pp. Chapt. 1;6
[3]	I.I. [I.I. Privalov] Priwalow, "Randeigenschaften analytischer Funktionen" , Deutsch. Verlag Wissenschaft. (1956) (Translated from Russian)
[4]	G.M. Goluzin, "Geometric theory of functions of a complex variable" , Transl. Math. Monogr. , 26 , Amer. Math. Soc. (1969) (Translated from Russian)
[5]	S.E. Warschawski, "On differentiability at the boundary in conformal mapping" Proc. Amer. Math. Soc. , 12 : 4 (1961) pp. 614–620
[6]	O.D. Kellogg, "Harmonic functions and Green's integral" Trans. Amer. Math. Soc. , 13 : 1 (1912) pp. 109–132
[7]	E.P. Dolzhenko, "Smoothness of harmonic and analytic functions at boundary points of a domain" Izv. Akad. Nauk SSSR Ser. Mat. , 29 (1965) pp. 1069–1084 (In Russian)