Conditional stability

of a point relative to a family of mappings

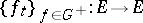Equicontinuity at this point of the family 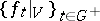of restrictions of the mappings 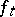to a certain manifold 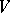imbedded in 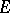(with the induced metric on 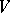); here 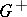is the set of real or integer non-negative numbers: 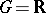or .

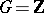The conditional stability of a point relative to a mapping is defined as the conditional stability relative to the family of non-negative powers of this mapping. The conditional stability of a point relative to a dynamical system 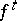is the conditional stability of this point relative to the family of mappings . The conditional stability of a solution of an equation

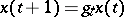given on 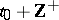is the conditional stability of the point 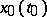relative to the family of mappings

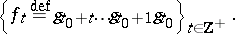The conditional stability of the solution 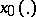of a differential equation

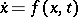(2)

given on 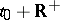is the conditional stability of the point 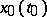relative to the family of mappings 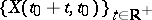, where is the Cauchy operator of this equation. The conditional stability of the solution 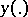of a differential equation of order ,

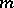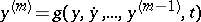given on 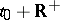, is the conditional stability of the solution 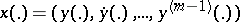, given on 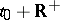, of the corresponding first-order differential equation of the form (2), where

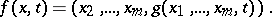The definitions 1)–5) below are some concrete examples of these and related notions.

1) Given a differential equation (2), where 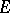is a normed -dimensional vector space and 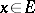. The solution 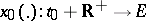of this equation is called conditionally stable with index 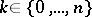if there is a 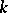-dimensional disc 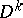imbedded in 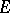(considered as a manifold of class 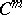), containing the point and having the following property: For each 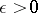there is a 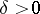such that for every 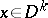satisfying the inequality 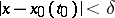, the solution of the same equation satisfying the initial condition is uniquely defined on 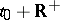, and for each 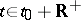satisfies the inequality 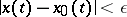. If the disc 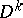with the given property may be chosen so that

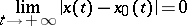(respectively,

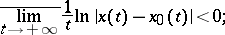here, and elsewhere is understood that 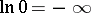) for every solution of the same equations starting in this disc (i.e. such that 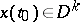), then the solution 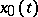is called asymptotically (respectively, exponentially) conditionally stable (with index ).

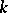The solution of the equation (2) ( or ) is called conditionally (asymptotically, exponentially conditionally) stable with index 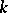, if it becomes such as the result of equipping 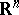(or 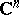) with a suitable norm. This property of the solution does not depend on the choice of norm.

2) Given an -dimensional Riemannian manifold 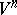(the distance function on which is written as 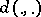), one calls a point 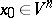conditionally stable (with index 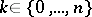) relative to a mapping 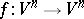if there is a (usually smooth) 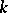-dimensional disc 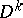imbedded in , containing the point and having the following property: For each 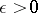there exists a such that for every satisfying the inequality 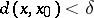, the inequality 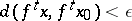holds for all 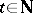. If the disc with the above property can be chosen so that

(respectively,

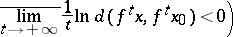for each 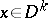, then the point 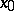is called asymptotically (respectively, exponentially) conditionally stable (with index 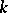) relative to the mapping .

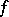Let be a compact differentiable manifold. A point is called conditionally stable (asymptotically, exponentially conditionally stable) with index 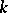relative to a mapping 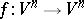if it becomes such as a result of equipping 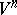with a suitable Riemannian metric. This property of does not depend on the choice of the Riemannian metric on .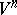

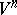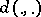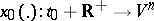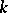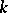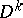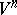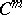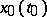3) Consider the differential equation (2) on an -dimensional Riemannian (or Finsler, cf. Finsler geometry) manifold , the distance function on which is denoted by . The solution of this equation is called conditionally stable (with index ) if there is a -dimensional disc imbedded in (considered as a manifold of class , where usually ), containing the point and having the following property: For each 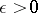there exists a 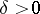such that for every satisfying the inequality , the solution 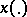of the same equation satisfying the initial condition is unique, defined on 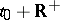, and for each satisfies the inequality 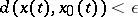. If the disc 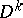with the above property may be taken so that

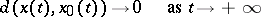(respectively,

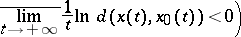for every solution of the same equation starting in this disc (i.e. such that 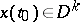), then the solution is called asymptotically (respectively, exponentially) conditionally stable (with index ).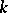

4) Let 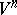be an -dimensional manifold of class 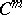and let be an open subset of it. Suppose that a point 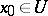is fixed under a family of mappings 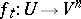of class 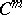(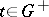, where 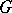is or 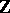). The fixed point 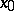is called conditionally stable (with index 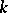) relative to the family of mappings if there is 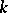-dimensional disc smoothly imbedded (by an imbedding of class ) in 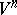such that for every neighbourhood 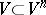of 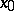there is a neighbourhood 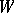of the same point such that 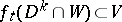for every . If the disc with this property may be taken so that 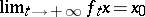for every 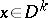, then the fixed point 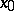is called asymptotically conditionally stable (with index 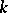) relative to the family of mappings .

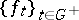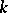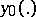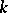5) The conditional (conditional asymptotic, conditional exponential) stability (with index ) of the solution of an equation of arbitrary order , is defined as the conditional (asymptotic, conditional exponential) stability (with index ) of the solution of the corresponding first-order equation (2), where

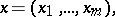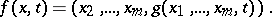Sometimes (cf. e.g. [3]) in defining conditional stability one requires the index to be non-zero: conditional stability with index zero always holds. Conditional stability (conditional asymptotic, conditional exponential stability) with index (the dimension of the phase space) is the same as Lyapunov stability (respectively, asymptotic, exponential stability).

The equilibrium positions under conditional stability have been investigated. Suppose that in a neighbourhood of a point an autonomous differential equation

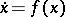(3)

is given, the right-hand side of which is continuously differentiable and vanishes at the point 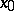. If in the open left half-plane in the complex plane there are 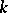eigen values of the derivative 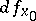, then this fixed point of equation (3) is conditionally exponentially stable with index 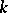(Lyapunov's theorem on conditional stability). For example, the upper equilibrium position 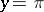, 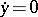of the equation of oscillation of a pendulum 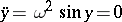is exponentially conditionally stable with index 1, because one of the roots of the characteristic equation 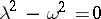of the variational equation (cf. Variational equations) 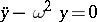is negative.

A fixed point 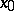of a differentiable mapping 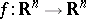is exponentially conditionally stable with index 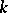relative to 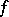if 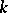eigen values of the derivative 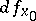lie in the open unit disc. A periodic point 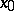of a differential mapping 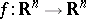, having period , is conditionally (asymptotically conditionally, exponentially conditionally) stable with index 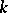relative to 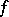if and only if it has this property relative to .

A periodic solution of an autonomous differential equation (3) with smooth right-hand side 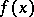having period 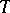is (asymptotically, exponentially) conditionally stable with index if and only if its value at the point 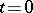is (respectively, asymptotically, exponentially) conditionally stable with index 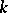relative to the mapping 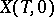, where 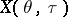is the Cauchy operator of (3).

The example of O. Perron (cf. Lyapunov stability) shows that the negativity of Lyapunov exponents of the variational equation along the solution of (3) does not imply the conditional stability with index 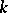of this solution. However, one has the following theorem, which shows that the situation described be Perron's example is not generic.

1) Let 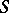be the set of all diffeomorphisms of a Euclidean space having uniformly continuous derivatives satisfying the inequality

For every diffeomorphism 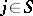denote by 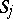the set of diffeomorphisms 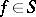satisfying the inequality

on the set 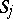the distance function

is given.

Fir each 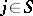one has in 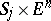an everywhere-dense set 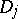of type 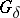with the following property: For every 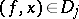the point is exponentially conditionally stable relative to the diffeomorphism 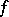with index

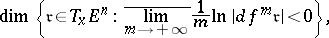i.e. with index equal to the number of negative Lyapunov characteristic exponents of the variational equation (cf. Lyapunov characteristic exponent).

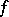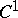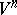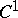2) For a dynamical system given on a closed differentiable manifold, analogous theorems can be formulated in a way that is more simple and invariant from the point of view of differential topology. Let be a closed differentiable manifold. The set of all diffeomorphisms of class mapping onto is equipped with the -topology. In the space 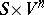there is an everywhere-dense set 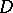of type with the following property: For each 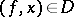the point is exponentially conditionally stable relative to the diffeomorphism 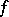with index

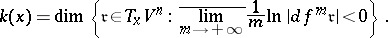(4)

3) For every diffeomorphism of a closed differentiable manifold 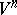and for every probability distribution on that is invariant relative to 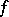(and the 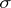-algebra of which contains all Borel sets), the set of points 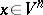that are exponentially conditionally stable with index (4) relative to 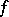has probability 1.

References

[1]	A.M. Lyapunov, "Collected works" , 2 , Moscow-Leningrad (1956) (In Russian)
[2]	B.F. Bylov, R.E. Vinograd, D.M. Grobman, V.V. Nemytskii, "The theory of Lyapunov exponents and its applications to problems of stability" , Moscow (1966) (In Russian)
[3]	B.P. Demidovich, "Lectures on the mathematical theory of stability" , Moscow (1967) (In Russian)
[4]	N.A. Izobov, "Linear systems of ordinary differential equations" J. Soviet Math. , 5 : 1 pp. 46–96 Itogi Nauk. i Tekhn. Mat. Anal. , 12 (1974) pp. 71–146
[5]	Ya.B. Pesin, "Characteristic Lyapunov exponents and smooth ergodic theory" Russian Math. Surveys , 32 : 4 (1977) pp. 55–114 Uspekhi Mat. Nauk , 32 : 4 (1977) pp. 55–112