Comparison function

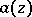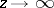A function that is used in studying the character of growth of the modulus of an entire function when ; a comparison is normally made here between the behaviour of and that of a certain "good" entire function . Here naturally arises the problem of describing a sufficiently broad set of entire functions the elements of which could successfully be used as "comparison standards" .

An entire function 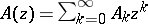is called a comparison function, or 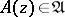, if: 1) 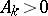(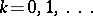); and 2) 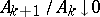as 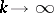. An entire function 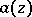is called -comparable if there exists a constant , , 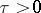such that

(1)

The lower bound 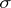of the numbers 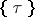for which the relation (1) is fulfilled is called the 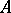-type of the -comparable entire function 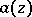. The following theorem on 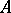-types holds: If an entire function 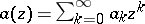is comparable with 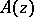, , 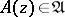then its 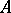-type 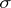can be calculated using the formula

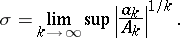(2)

The given class 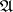of comparison functions is known to give a complete solution of this problem, since for any entire function , other than a polynomial, there exists a comparison function , , such that 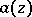is comparable with 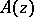and such that its 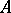-type is equal to 1.

If an entire function is comparable with 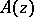, , and its -type is equal to 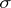, then the function

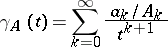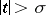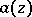is analytic, according to (2), for ; it is called -associated with . In this case, the generalized Borel representation holds for :

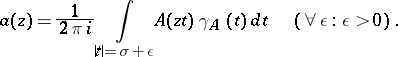(3)

If 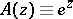is taken as a comparison function, then (3) is the classical Borel integral representation of entire functions of exponential type .

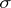If 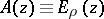holds in (3), where 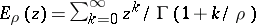(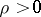) is a Mittag-Leffler function, then (3) is an integral representation for any entire function of order 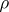and of type 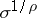( 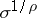is the type of 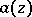in the classical sense).

For certain cases of , an inverse transformation to (3) has been constructed (see, for example [1], which has a bibliography relating to comparison functions). Comparison functions and the Borel representation (3) are used in various questions of analysis (see, for example, [2], [3]). If 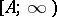is the class of entire functions that are comparable with a given comparison function 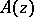, then, for any sequence of comparison functions , there always exists an entire function such that .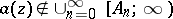

References

[1]	R.P. Boas, R.C. Buck, "Polynomial expansions of analytic functions" , Springer & Acad. Press (U.S.A. & Canada) (1958)
[2]	M.M. Dzhrbashyan, "Integral transforms and representation of functions in the complex domain" , Moscow (1966) (In Russian)
[3]	Yu.A. Kaz'min, "A certain problem of A.O. Gel'fond" Math. USSR Sb. , 19 : 4 (1973) pp. 509–530 Mat. Sb. , 90 : 4 (1973) pp. 521–543