Cohomological dimension

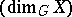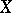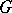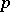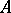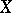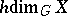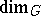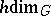The cohomological dimension of a topological space relative to the group of coefficients is the maximum integer for which there exists closed subsets of such that the cohomology groups are non-zero. The homological dimension is similarly defined (cf. Homological dimension of a space). Finite Lebesgue dimension (covering dimension) is the same as (or ) if 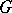is the subgroup of the integers (or real numbers modulo 1). In Euclidean space 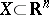the equation 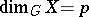is equivalent to the property that 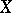is locally linked by -dimensional cycles (with coefficients in 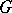). 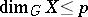For paracompact spaces , the inequality is equivalent to the existence of soft resolutions (cf. Soft sheaf and Resolution) for 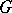of length 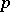. Since soft sheaves are acyclic, in this way a connection is established with the general definition of dimension in homological algebra; for example, the injective (or projective) dimension of a module is 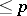if it has an injective (or projective) resolution of length 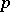; the global dimension of a ring is the maximum of the injective (or projective) dimensions of the modules over the ring and is the analogue of the Lebesgue dimension of .

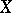References

[1]	P.S. Aleksandrov, Ann. of Math. (1929) pp. 101–187 , 30
[2]	P.S. Aleksandrov, "Dimensionstheorie: Ein Beitrag zur Geometrie der abgeschlossenen Mengen" Math. Ann. , 106 (1932) pp. 161–238
[3]	P.S. Aleksandrov, "An introduction to homological dimension theory and general combinatorial topology" , Moscow (1975) (In Russian)
[4]	A.E. [A.E. Kharlap] Harlap, "Local homology and cohomology, homology dimension and generalized manifolds" Math. USSR Sb. , 25 : 3 (1975) pp. 323–349 Mat. Sb. , 96 : 3 (1975) pp. 347–373 Zbl 0325.57002
[5]	V.I. Kuz'minov, "Homological dimension theory" Russian Math. Surveys , 29 : 5 (1968) pp. 1–45 Uspekhi Mat. Nauk , 23 : 5 (1968) pp. 3–49 Zbl 0187.20103
[6]	G.E. Bredon, "Sheaf theory" , McGraw-Hill (1967) MR0221500 Zbl 0158.20505
[7]	H. Cartan, S. Eilenberg, "Homological algebra" , Princeton Univ. Press (1956) MR0077480 Zbl 0075.24305

E.G. Sklyarenko

The cohomological dimension of a scheme is the analogue of the notion of the cohomological dimension of a topological space for an algebraic variety or a scheme with a selected cohomology theory. Let 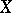be an algebraic variety or a Noetherian scheme of dimension . The cohomological dimension of 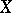is defined to be the integer 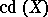equal to the infimum of all those 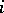for which 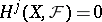for all Abelian sheaves 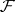on the topological space 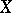when 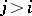. The inequality

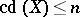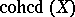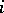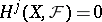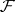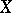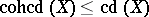holds. The coherent cohomological dimension of the scheme is the number equal to the infimum of those for which for all coherent algebraic sheaves (cf. Coherent algebraic sheaf) on when . By definition, . By Serre's theorem, 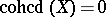if and only if 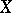is an affine scheme. On the other hand, if 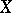is an algebraic variety over a field , then 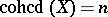if and only if 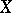is proper over 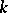(Lichtenbaum's theorem, see [3]).

Let 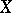be a proper scheme over a field 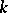, let 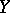be a closed subscheme of 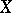of codimension and let 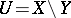. Then the following statements hold ([2]–[4]).

If is the set-theoretic complete intersection of ample divisors on 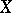, then

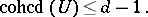If 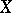is a projective Cohen–Macaulay variety (for instance, a non-singular projective variety) and 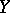is zero-dimensional, then 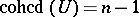. The condition 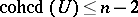is equivalent to 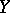being connected. If 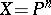is a projective space and 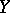is connected and has dimension 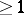, then

If is a complex algebraic variety, then one can consider the cohomological dimension of the corresponding topological space 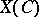. In the general case when 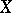is a Noetherian scheme, the analogue of cohomological dimension is the notion of the étale cohomological dimension of the scheme 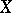. More precisely, let 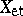be the étale topology of the Grothendieck scheme 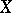and let be a prime number. 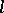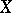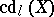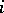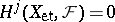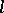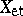By the cohomological -dimension of the scheme (or the étale cohomological dimension) one means the number equal to the infimum of those for which for all -torsion Abelian sheaves on when . If is an affine scheme, then is also called the cohomological dimension of the ring . 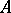In particular, if is a field, then the notion of is the same as that of the cohomological dimension of a field as studied in the theory of Galois cohomology.

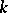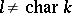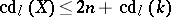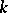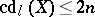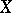If is an algebraic variety of dimension over a field and if , then . In particular, if is a separably closed field, then . If is an affine algebraic variety over the separably closed field 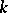, then .

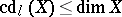Let 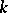be a field of finite characteristic 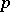; then for any Noetherian scheme 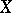over , the inequality

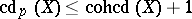holds. In particular, for any Noetherian commutative ring ,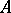

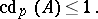If is a quasi-projective algebraic variety over the separably closed field , then 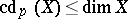, where is the characteristic of .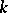

References

[1]	A. Grothendieck, "Sur quelques points d'algèbre homologique" Tohôku Math. J. , 9 (1957) pp. 119–221 MR0102537
[2]	R. Hartshorne, "Cohomological dimension of algebraic varieties" Ann. of Math. , 88 (1968) pp. 403–450 MR0232780 Zbl 0169.23302
[3]	R. Hartshorne, "Ample subvarieties of algebraic varieties" , Springer (1970) MR0282977 Zbl 0208.48901
[4]	R. Hartshorne, "Cohomology of non-complete algebraic varieties" Compositio Math. (1971) pp. 257–264 MR0302649 Zbl 0221.14014
[5]	M. Artin (ed.) A. Grothendieck (ed.) J.-L. Verdier (ed.) , Théorie des topos et cohomologie étale des schemas (SGA 4, vol. II, III) , Lect. notes in math. , 270; 305 , Springer (1972–1973) MR1080173 MR1080172 MR0717602 MR0717586 MR0505104 MR0505101

I.V. Dolgachev

References

[a1]	B. Iversen, "Cohomology of sheaves" , Springer (1986) MR0842190 Zbl 0559.55001