Characterization theorems

in probability theory and mathematical statistics

Theorems that establish a connection between the type of the distribution of random variables or random vectors and certain general properties of functions in them.

Example 1.

Let be a three-dimensional random vector such that:

1) its projections 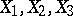onto any three mutually-orthogonal axes are independent; and

2) the density 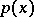, , 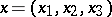of the probability distribution of 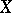depends only on 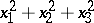. Then the distribution of 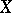is normal and

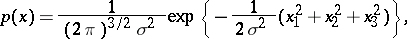where 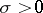is a certain constant (the Maxwell law for the distribution of the velocities of molecules in a gas in stationary state).

Example 2.

Let 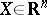be a random vector with independent and identically-distributed components . If the distribution is normal then the "sample meansample mean"

and the "sample variancesample variance"

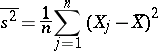are independent random variables. Conversely, if they are independent, then the distribution of 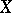is normal.

Example 3.

Let 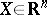be a vector with independent and identically-distributed components. There are non-zero constants , such that the random variables

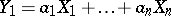and

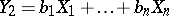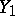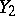are independent if and only if has a normal distribution. The last assertion remains true if the assumption that and are independent is replaced by the assumption that they are identically distributed, adding, however, certain restrictions on the coefficients 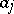and .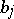

A characterization of a similar kind of the distribution of a random vector 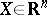by the property of identical distribution or of independence of two polynomials 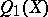and 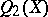is given by a number of characterization theorems that play an important role in mathematical statistics.

References

[1]	A.M. Kagan, Yu.V. Linnik, S.R. Rao, "Characterization problems in mathematical statistics" , Wiley (1973) (Translated from Russian)