Chaplygin theorem
on differential inequalities
If in the differential inequality
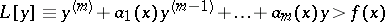 | (*) |
all the  and
and 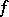 are summable on
are summable on 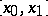 , then there exists an
, then there exists an  , independent of
, independent of 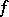 , such that
, such that 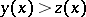 ,
, 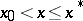 , where
, where
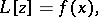 |
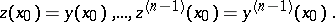 |
Here
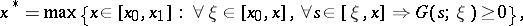 |
where 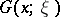 is the corresponding Cauchy function, i.e. the solution of the equation
is the corresponding Cauchy function, i.e. the solution of the equation 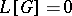 ,
, 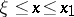 , that satisfies the initial conditions
, that satisfies the initial conditions
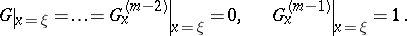 |
Thus, for  , and also for the inequality
, and also for the inequality  , one obtains
, one obtains 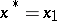 , while for the inequality
, while for the inequality 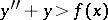 one obtains
one obtains
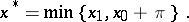 |
Analogous statements hold: for weak inequalities; for the comparison of 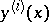 with
with 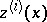 ,
, 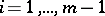 ; for initial conditions of the form
; for initial conditions of the form
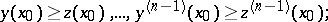 |
and for solutions of the inequality (*) with 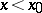 .
.
The theorem was obtained by S.A. Chaplygin in 1919.
See also the references in Differential inequality.
References
| [1] | Ya.D. Mamedov, S. Ashirov, S. Atdaev, "Theorems on inequalities" , Ashkhabad (1980) (In Russian) |
Comments
On page 123 of [a1] Chaplygin's theorem is formulated as a problem.
References
| [a1] | I.G. Petrovskii, "Ordinary differential equations" , Prentice-Hall (1966) (Translated from Russian) |
Chaplygin theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Chaplygin_theorem&oldid=46310