Chain space
An incidence system to axiomatically describe chain geometries (cf. Chain geometry).
A weak chain space is an incidence system 
satisfying the three conditions below. Here, the elements of 
are called chains and two different points (i.e., elements of 
) are called distant if they are incident with a common chain.
i) any three pairwise distant points are contained in exactly one chain;
ii) any chain contains at least three points;
iii) any point lies in at least one chain.
For a point 
, let 
be the set of all points distant to 
and let 
. Then the incidence system 
is called the residual space of 
at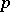 
.
A partial parallel structure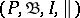 
is an incidence system 
together with an equivalence relation 
on 
satisfying the two conditions below. Here, the elements of 
are called lines.
a) two different points are incident with at most one line;
b) for a line 
and point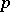 
, there is exactly one line, 
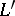, incident with 
and such that 
. Condition b) is the Euclid parallel axiom.
A partial parallel structure 
is called a partial affine space if there is an affine space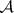 
such that 
is the set of points of 
, 
is the set of straight lines of 
and 
is the natural parallelism on 
.
A weak chain space is called a chain space if all residual spaces of it are partial affine spaces.
Every proper chain geometry is a chain space. Conversely, the chain spaces that are proper chain geometries can be characterized by suitable automorphism groups [a1].
A contact space 
is a weak chain space 
together with a family 
, where 
is an equivalence relation on 
with the following properties:
1) if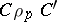 
, then 
is the only point common to 
and 
;
2) if 
and 
is a point distant to 
, then there is a unique chain 
incident with 
and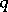 
for which 
.
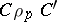Clearly, for a contact space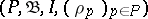 
any residual space of the incidence system 
gives rise to a partial parallel structure 
. Conversely, any chain space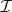 
is a contact space (taking for 
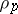the natural parallelism of the affine space underlying 
). One can characterize the contact spaces that are chain spaces by certain configurations together with richness conditions [a3].
An affine chain space 
is a contact space, where 
is the point set of an affine space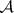 
. The elements of 
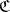are called affine chains and are normal rational curves in 
, i.e., affine parts of curves which are a Veronese variety (cf. Veronese mapping). For the set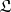 
of all affine chains that are straight lines, the structure 
is a partial affine space. Affine chain spaces can be constructed by means of Jordan algebras [a2] (cf. also Jordan algebra).
A classical example is the quadric model of a chain space, constructed on a quadric 
by means of plane sections. Moreover, the stereographic projection from a simple point 
of 
(to a hyperplane different from the tangent plane of 
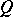at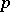 
) then gives rise to an affine chain space (cf. also Benz plane).
References
| [a1] | A. Herzer, "Chain geometries" F. Buekenhout (ed.) , Handbook of Incidence Geometry , North-Holland (1995) |
| [a2] | A. Herzer, "Affine Kettengeometrien über Jordan-Algebren" Geom. Dedicata , 59 (1996) pp. 181–195 |
| [a3] | S. Meuren, A. Herzer, "Ein Axiomsystem für partielle affine Räume" J. Geom. , 50 (1994) pp. 124–142 |
Chain space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Chain_space&oldid=46302