Bounded operator
A mapping 
of a topological vector space 
into a topological vector space 
such that 
is a bounded subset in 
for any bounded subset 
of 
. Every operator 
, continuous on 
, is a bounded operator. If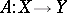 
is a linear operator, then for 
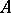to be bounded it is sufficient that there exists a neighbourhood 
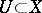such that 
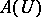is bounded in 
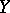. Suppose that 
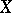and 
are normed linear spaces and that the linear operator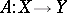 
is bounded. Then
 |
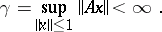This number is called the norm of the operator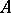 
and is denoted by 
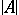. Then
 |
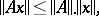and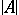 
is the smallest constant 
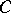such that
 |
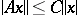for any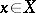 
. Conversely, if this inequality is satisfied, then 
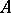is bounded. For linear operators mapping a normed space 
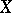into a normed space 
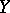, the concepts of boundedness and continuity are equivalent. This is not the case for arbitrary topological vector spaces 
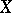and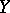 
, but if 
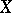is bornological and 
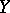is a locally convex space, then the boundedness of a linear operator 
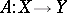implies its continuity. If 
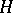is a Hilbert space and 
is a bounded symmetric operator, then the quadratic form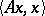 
is bounded on the unit ball 
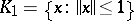. The numbers
 |
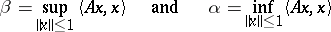are called the upper and lower bounds of the operator 
. The points 
and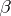 
belongs to the spectrum of 
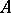, and the whole spectrum lies in the interval 
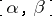. Examples of bounded operators are: the projection operator (projector) onto a complemented subspace of a Banach space, and an isometric operator acting on a Hilbert space.
If the space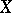 
and 
have the structure of a partially ordered set, for example are vector lattices (cf. Vector lattice), then a concept of order-boundedness of an operator can be introduced, besides the topological boundedness considered above. An operator 
is called order-bounded if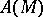 
is an order-bounded set in 
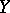for any order-bounded set 
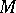in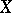 
. Examples: an isotone operator, i.e. an operator such that 
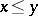implies 
.
References
| [1] | L.A. Lyusternik, V.I. Sobolev, "Elemente der Funktionalanalysis" , Akademie Verlag (1955) (Translated from Russian) |
| [2] | W. Rudin, "Functional analysis" , McGraw-Hill (1973) |
| [3] | G. Birkhoff, "Lattice theory" , Colloq. Publ. , 25 , Amer. Math. Soc. (1967) |
Comments
References
| [a1] | A.E. Taylor, D.C. Lay, "Introduction to functional analysis" , Wiley (1980) |
| [a2] | A.C. Zaanen, "Riesz spaces" , II , North-Holland (1983) |
Bounded operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Bounded_operator&oldid=46139