Boundary value problem, elliptic equations

The problem of finding a solution , regular in a domain , to an elliptic equation

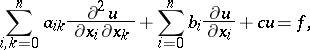(1)

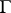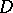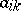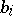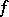which satisfies certain additional conditions on the boundary of . Here , , and are given functions on .

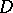The classical boundary value problems are special cases of the following problem: Find a solution to equation (1), regular in a domain 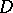and satisfying on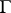

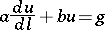(2)

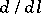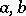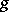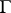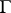where denotes differentiation in some direction, and and are given continuous functions on with everywhere on (see [1]).

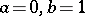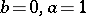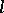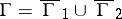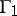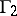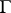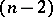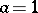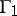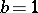Putting , one obtains the Dirichlet problem; with one has a problem with oblique derivative (see Differential equation, partial, oblique derivatives), which becomes a Neumann problem if is the direction of the conormal. If , where and are disjoint open subsets of , and is either empty or an -dimensional manifold, with , on , , on 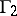, one obtains a mixed problem.

Problem (2) has been studied for elliptic equations in two independent variables (see [2]). Fairly complete investigations have been made of the Dirichlet problem for elliptic equations in any finite number of independent variables (see [1], [3], [4]) and the problem with oblique derivative in case the direction 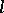is not contained in a tangent plane to 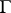at any point of 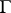. In that case the problem with oblique derivative is a Fredholm problem and the solution is smooth to the same order as the field of directions 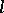and the function 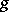(see [1]). The case in which 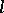lies in a tangent plane to 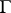at certain points of 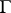has been studied (see [3]). The local properties of solutions to the problem with oblique derivative have been investigated (see [5]). At points where the field 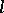lies in a tangent plane to , the solution of the problem is less smooth than 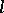and 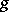. This has been used as a basis for investigating the problem in a generalized setting (see [7], [8]).

Consider the following boundary problem for harmonic functions regular in the unit ball :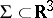

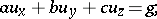let 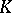be the set of points of the unit sphere 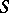at which the function vanishes. The vector field 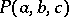lies in a tangent plane to 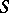at the points of . Suppose in addition that 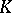is the union of a finite number of disjoint curves; let 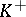be the subset of 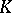consisting of those points at which 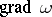makes an acute angle with the projection of the field 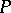on 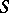, and let 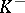be the remaining part of 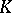. A generalized formulation of the problem is obtained when the values of are also prescribed on 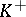, whereas on 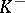the solution is allowed to have integrable singularities. If 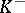is empty, the solution to the generalized problem may be made arbitrarily smooth by increasing the smoothness of the additional data of the problem. Generally speaking, a solution to the mixed problem on the set 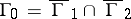has singularities (see [1]). In order to eliminate such singularities on 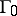, one must impose additional conditions on the data (see [11]).

A large category of boundary value problems is constituted by what are known as problems with free boundaries. In these problems one must find not only a solution of equation (1), but also the domain in which it is regular. The boundary 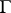of the domain is unknown, but two boundary conditions must be satisfied on it. An example of this type of problem is the problem of wave motions of an ideal fluid: Find a harmonic function , regular in some domain 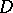, where part of the boundary, 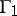say, is known and the normal derivative 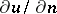is given on 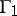; the other part of the boundary, 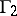, is unknown and on it one gives two boundary conditions:

where 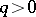is a given function.

For harmonic functions of two independent variables, one uses conformal mapping (see [12], [13], [14]). See also Differential equation, partial, free boundaries.

The following problem has been investigated: Find a harmonic function , regular in a domain 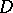and satisfying the condition

where 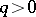is a given function, on the boundary 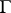. There is a complete solution of this problem for harmonic functions of two independent variables (see [14]).

Given an equation 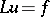, where 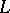is an operator of order 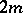, uniformly elliptic in the closure 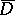of a domain 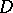, consider the problem of determining a solution , regular in 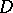and satisfying on the boundary 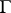of 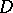the conditions

(3)

where 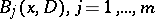, are differential operators satisfying the following complementarity condition.

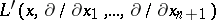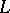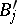Let be the principal part of , let be the principal part of 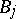, the normal to 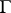at a point and 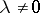an arbitrary vector parallel to 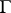. Let 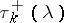denote the roots of 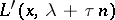with positive imaginary parts. The polynomials 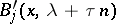, , 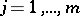as functions of , must be linearly independent modulo the polynomial 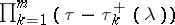. In this case, too, the problem is normally solvable. Violation of the complementarity condition may entail an essential change in the nature of the problem (see [17]).

Problem (2) is a special case of problem (3). For problem (2) with 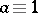, the complementarity condition is equivalent to the condition that there be no point on the boundary of the domain at which the direction 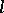lies in a tangent plane to the boundary.

Another particular case of problem (3) is the boundary value problem

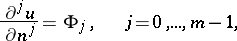which is an analogue, to some extent, of the Dirichlet problem for higher-order elliptic equations.

The boundary value problem has been studied for the poly-harmonic equation 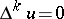when the boundary of the domain consists of manifolds of different dimensions (see [15]).

In investigations of boundary value problems for non-linear equations (e.g. the Dirichlet and Neumann problems), much importance attaches to a priori estimates, various fixed-point principles (see [17], [18]) and the generalization of Morse theory to the infinite-dimensional case (see [19]).

References

[1]	C. Miranda, "Partial differential equations of elliptic type" , Springer (1970) (Translated from Italian) MR0284700 Zbl 0198.14101
[2]	I.N. Vekua, "Generalized analytic functions" , Pergamon (1962) (Translated from Russian) MR0152665 MR0150320 MR0138774 Zbl 0127.03505 Zbl 0100.07603
[3]	A.V. Bitsadze, "Boundary value problems for second-order elliptic equations" , North-Holland (1968) (Translated from Russian) MR0226183 Zbl 0167.09401
[4]	M.V. Keldysh, "On the solvability and stability of the Dirichlet problem" Uspekhi Mat. Nauk : 8 (1941) pp. 171–231 (In Russian) Zbl 0179.43901
[5]	L. Hörmander, "Pseudo-differential operators and non-elliptic boundary value problems" Ann. of Math. , 83 : 1 (1966) pp. 129–209 MR233064
[6]	R.L. Borrelli, "The singular, second order oblique derivative problem" J. Math. and Mech. , 16 : 1 (1966) pp. 51–81 MR0203217 Zbl 0143.14603
[7]	Yu.V. Egorov, V.A. Kondrat'ev, "The oblique derivative problem" Mat. Sb. , 78 : 1 (1969) pp. 148–176 (In Russian) MR0237953 Zbl 0186.43202 Zbl 0165.12202
[8]	V.G. Maz'ya, "The degenerate problem with oblique derivative" Mat. Sb. , 87 : 3 (1972) pp. 417–453 (In Russian)
[9]	A. Yanushauskas, Dokl. Akad. Nauk SSSR , 164 : 4 (1965) pp. 753–755
[10]	M.I. Vishik, G.I. Eskin, "Sobolev–Slobodinsky spaces of variable order with weighted norm, and their applications to mixed boundary value problems" Sibirsk. Mat. Zh. , 9 : 5 (1968) pp. 973–997 (In Russian)
[11]	G. Giraud, Ann. Soc. Math. Polon. , 12 (1934) pp. 35–54
[12]	M.A. Lavrent'ev, "Variational methods for boundary value problems for systems of elliptic equations" , Noordhoff (1963) (Translated from Russian) Zbl 0121.06701
[13]	A.I. Nekrasov, "Exact theory of waves of stationary type on the surface of a heavy fluid" , Collected works , 1 , Moscow (1961) (In Russian)
[14]	F.D. Gakhov, "Boundary value problems" , Pergamon (1966) (Translated from Russian) MR0198152 Zbl 0141.08001
[15]	S.L. Sobolev, Mat. Sb. , 2 : 3 (1937) pp. 465–499
[16]	S. Agmon, A. Douglis, L. Nirenberg, "Estimates near the boundary for solutions of elliptic partial differential equations satisfying general boundary conditions I, II." Comm. Pure Appl. Math. , 17 (1964) pp. 35–92 MR162050
[17]	J. Schauder, Math. Z. , 33 (1931) pp. 602–640
[18]	J. Leray, J. Schauder, Ann. Sci. Ecole Norm. Sup. Ser. 3 , 51 (1934) pp. 45–78
[19]	R.S. Palais, "Morse theory on Hilbert manifolds" Topology , 2 : 4 (1963) pp. 299–340 MR0158410 Zbl 0122.10702

References

[a1]	R. Courant, D. Hilbert, "Methods of mathematical physics. Partial differential equations" , 2 , Interscience (1965) (Translated from German) MR0195654
[a2]	P.R. Garabedian, "Partial differential equations" , Wiley (1964) MR0162045 Zbl 0124.30501
[a3]	A. Friedman, "Partial differential equations" , Holt, Rinehart & Winston (1969) MR0445088 Zbl 0224.35002