Boks integral

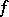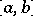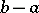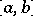One of the generalizations of the Lebesgue integral, proposed by A. Denjoy (1919) and studied in detail by T.J. Boks (1921). A real-valued function on a segment is periodically extended (with period length ) to the entire straight line. For an arbitrary subdivision of , for an arbitrary selection of points 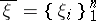, , 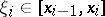and an arbitrary 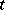, the following sum is constructed:

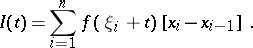If, for 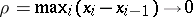, 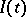converges in measure to a definite limit 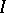, the number 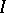is said to be the Boks integral (-integral) of over . Thus, the Boks integral is an integral of Riemann type and is a generalization of the Riemann integral.

The Boks integral represents a considerable extension of the Lebesgue integral: Any summable function is 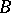-integrable and these integrals coincide, but there exist non-summable 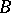-integrable functions; in particular, if 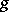is the function conjugate with a summable function 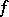, then it is 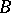-integrable and the coefficients of the series conjugate with the Fourier series of 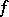are the coefficients of the respective Fourier series (in the sense of 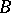-integration) of (A.N. Kolmogorov). The theory of the Boks integral was not further developed, since the -integral proved to be more convenient for the integration of functions conjugate with summable functions.

References

[1]	T.J. Boks, "Sur les rapports entre les méthodes de l'intégration de Riemann et de Lebesque" Rend. Circ. Mat. Palermo (2) , 45 (1921) pp. 211–264
[2]	A. Zygmund, "Trigonometric series" , 1–2 , Cambridge Univ. Press (1988)