Bogolyubov inequality

in statistical mechanics

Bogolyubov's inequality for the free-energy functional is an inequality that gives rise to a variational principle of statistical mechanics. The following inequality is valid for any Hermitian operators 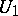and :

(*)

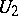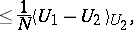where

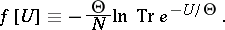This expression has the meaning of the free-energy density for a system with Hamiltonian 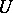; the extensive parameter is the number of particles or the volume, depending on the system; 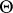is the absolute temperature in energy units, and

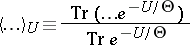denotes the thermodynamic average with respect to the Hamiltonian .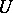

The Bogolyubov inequality (*) is used to obtain the exact thermodynamic limit solutions of model problems in statistical quantum physics [1], [2], in studies using the method of molecular fields [3], in proving the existence of the thermodynamic limit, and also in order to obtain physically important estimates for the free energies of various multi-particle systems [4]. There exist generalizations of the Bogolyubov inequality (*) to the case of von Neumann algebras with a "trace" [5] and general von Neumann algebras [6].

References

[1]	N.N. Bogolyubov, jr., "On model dynamical systems in statistical mechanics" Physica , 32 (1966) pp. 933–944
[2]	N.N. Bogolyubov jr., "A method for studying model Hamiltonians" , Pergamon (1972) (Translated from Russian)
[3]	S.V. Tyablikov, "Methods of the quantum theory of magnetism" , Plenum (1967) (Translated from Russian)
[4]	L.P. Kudrin, "Statistical plasma physics" , Moscow (1974) (In Russian)
[5]	M.B. Ruskai, "Inequalities for traces on von Neumann algebras" Comm. Math. Phys. , 26 (1972) pp. 280–289
[6]	H. Araki, "Golden–Thompson and Peierls–Bogolyubov inequalities for a general von Neumann algebra" Comm. Math. Phys. , 34 (1973) pp. 167–178

References

[a1]	D. Ruelle, "Statistical mechanics: rigorous results" , Benjamin (1974)

Bogolyubov's inequality for Green functions and correlation functions. The following inequality holds for time-temperature commutator Green functions (cf. Green function in statistical mechanics). If one defines

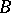where is the operator in the Heisenberg representation for imaginary time 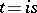, denotes the thermodynamic average for Hamiltonian 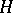and 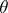is the absolute temperature, then

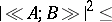(1)

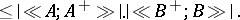Substituting one gets

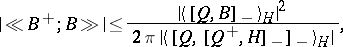(2)

where 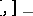is the commutator. One may also write down the inequality

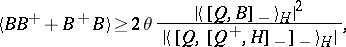(3)

which follows from (2). Owing to the general nature of the inequalities (2) and (3) they are extensively employed in studies of various physical systems.

An improved estimate of the correlation function

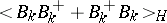is attained in (3) by selecting as the operator 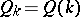some "quasi-integral" of motion which, for 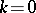, commutes with the Hamiltonian 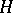. The commutator in the numerator of the right-hand side of (3) describes the transformation properties of under the infinitesimal transformations of the continuous symmetry group generated by the operator 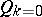. The inequalities (2) and (3) are effectively employed in the study of systems with a spontaneous symmetry breaking: In such a case thermodynamic averages must be considered in the framework of the method of quasi-averages (cf. Quasi-averages, method of).

Similar inequalities are applicable to Green functions in classical statistical mechanics, where the respective commutators "become" Poisson brackets.

Bogolyubov's inequalities made it possible to establish several relations concerning model systems of statistical physics, to study the problem of ordering in infinite systems, etc. For references see Bogolyubov theorem.