Biharmonic function

A function 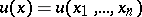of real variables, defined in a domain of the Euclidean space , , with continuous partial derivatives up to the fourth order inclusive, that satisfies in the equation

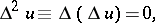where is the Laplace operator. This equation is known as the biharmonic equation. The class of biharmonic functions includes the class of harmonic functions and is a subclass of the class of poly-harmonic functions (cf. Harmonic function; Poly-harmonic function). Each biharmonic function is an analytic function of the coordinates .

From the point of view of practical applications the biharmonic functions in two variables 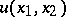are the most important. Such biharmonic functions can be represented using harmonic functions or 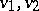, in the form

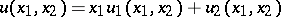or

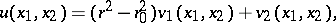where 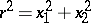, while 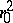is a constant. The main boundary value problem for biharmonic functions is the following: To find a biharmonic function in the domain 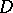that is continuous together with its first-order derivatives in the closed domain and satisfies on the boundary 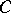the conditions

(*)

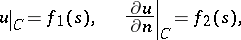where 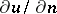is the derivative with respect to the normal on , and 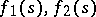are given continuous functions of the arc length on the contour . The above representations of biharmonic functions give the solution of problem (*) in an explicit form for the case of the disc , starting from the Poisson integral for harmonic functions [1].

Biharmonic functions in two variables may also be represented as follows:

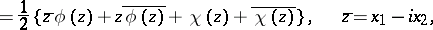using two analytic functions 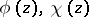of the complex variable 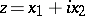. This representation makes it possible to reduce the boundary value problem (*) for an arbitrary domain to a system of boundary value problems for analytic functions, for which a method of solving was developed in detail by G.V. Kolosov and N.I. Muskhelishvili. This method was developed while solving various planar problems of elasticity theory (cf. Elasticity theory, planar problem of), in which the main biharmonic function is the stress function or the Airy function [2], [3] (cf. Airy functions).

References

[1]	A.N. [A.N. Tikhonov] Tichonoff, A.A. Samarskii, "Differentialgleichungen der mathematischen Physik" , Deutsch. Verlag Wissenschaft. (1959) (Translated from Russian)
[2]	N.I. Muskhelishvili, "Some basic problems of the mathematical theory of elasticity" , Noordhoff (1975) (Translated from Russian)
[3]	M.A. Lavrent'ev, B.V. Shabat, "Methoden der komplexen Funktionentheorie" , Deutsch. Verlag Wissenschaft. (1967) (Translated from Russian)

Comments

An axiomatic treatment of biharmonic functions, similar to that of harmonic functions, is given in [a1], [a2].

References

[a1]	E.P. Smyrnelis, "Axiomatique des fonctions biharmoniques" Ann. Inst. Fourier (Grenoble) , 25 : 1 (1975) pp. 35–97
[a2]	E.P. Smyrnelis, "Axiomatique des fonctions biharmoniques" Ann. Inst. Fourier (Grenoble) , 26 : 3 (1976) pp. 1–47