Best approximations, sequence of

A sequence , of numbers, where is the best approximation of an element of a normed linear space by elements of an -dimensional subspace , with , so that . Usually, 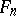is the linear span of the first elements of some fixed system 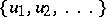of linearly independent elements of .

In the case 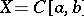and is the subspace of algebraic polynomials of degree 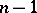, sequences of best approximations were first considered in the 1850s by P.L. Chebyshev; the fact that

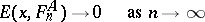for any function 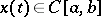was established in 1885 by K. Weierstrass. In the general case, the relation

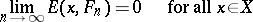is always satisfied when the union of the subspaces 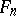, 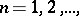is everywhere dense in ,

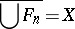(essentially, this is an equivalent statement). However, the sequence may converge to zero arbitrarily slowly. This follows from a theorem of Bernstein: If 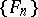is a sequence of subspaces of dimension of a normed linear space 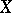, such that 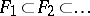and 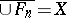, then, for any monotone decreasing null sequence 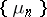of non-negative real numbers, there exists an such that 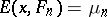, . In the function spaces 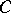and 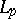, the rate at which a sequence of best approximations tends to zero depends both on the system of subspaces 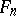and on the smoothness characteristics of the approximated function (the modulus of continuity, the existence of derivatives up to a specific order, etc.), and it can be estimated in terms of these characteristics. Conversely, knowing the rate of convergence to zero of the sequence , one can draw conclusions with respect to the smoothness of (see Approximation of functions, direct and inverse theorems).

References

[1]	S.N. Bernshtein, "Collected works" , 2 , Moscow (1954) (In Russian)
[2]	V.L. Goncharov, "The theory of interpolation and approximation of functions" , Moscow (1954) (In Russian)
[3]	A.F. Timan, "Theory of approximation of functions of a real variable" , Pergamon (1963) (Translated from Russian)

Comments

Theorems inferring smoothness characteristics of a function 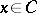or 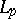from properties of were first given by D. Jackson in 1911 for algebraic or trigonometric approximation, see Jackson theorem. Theorems converse to these, i.e. inferring properties of 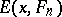from smoothness characteristics of the function , have been proved by S.N. Bernstein [S.N. Bernshtein] and A. Zygmund, cf. Bernstein theorem. See also [a2], Chapt. 4, Sect. 6 and Chapt. 6, Sect. 3.

References

[a1]	I.P. Natanson, "Constructive function theory" , 1–3 , F. Ungar (1964–1965) (Translated from Russian)
[a2]	E.W. Cheney, "Introduction to approximation theory" , Chelsea, reprint (1982) pp. 203ff