Bessel inequality

The inequality

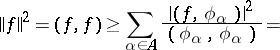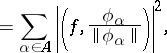where is an element of a (pre-) Hilbert space with scalar product and is an orthogonal system of non-zero elements of . The right-hand side of Bessel's inequality never contains more than a countable set of non-zero components, whatever the cardinality of the index set . Bessel's inequality follows from the Bessel identity

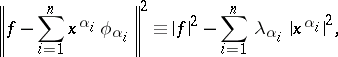which is valid for any finite system of elements 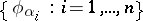. In this formula the are the Fourier coefficients of the vector with respect to the orthogonal system , i.e.

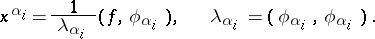The geometric meaning of Bessel's inequality is that the orthogonal projection of an element on the linear span of the elements , , 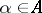has a norm which does not exceed the norm of (i.e. the hypothenuse in a right-angled triangle is not shorter than one of the other sides). For a vector to belong to the closed linear span of the vectors , , it is necessary and sufficient that Bessel's inequality becomes an equality. If this occurs for any 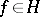, one says that the Parseval equality holds for the system in .

For a system of linearly independent (not necessarily orthogonal) elements of Bessel's identity and Bessel's inequality assume the form

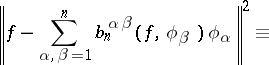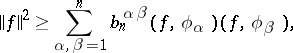where are the elements of the matrix inverse to the Gram matrix (cf. Gram determinant) of the first vectors of the initial system.

The inequality was derived by F.W. Bessel in 1828 for the trigonometric system.

References

[1]	L.D. Kudryavtsev, "Mathematical analysis" , 2 , Moscow (1973) (In Russian)

Comments

Usually, the orthogonal system of elements is orthonormalized, i.e. one sets 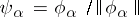. Bessel's inequality then takes the form

which is easier to remember. In this form it is used in approximation theory, Fourier analysis, the theory of orthogonal polynomials, etc.

References

[a1]	K. Yosida, "Functional analysis" , Springer (1980) pp. Chapt. 8, Sect. 4; 5
[a2]	E.W. Cheney, "Introduction to approximation theory" , Chelsea, reprint (1982) pp. 203ff
[a3]	P.J. Davis, "Interpolation and approximation" , Dover, reprint (1975) pp. 108–126
[a4]	E. Hewitt, K.R. Stromberg, "Real and abstract analysis" , Springer (1965)