Bendixson sphere
The sphere in real analysis which is known as the Riemann sphere in the theory of functions of a complex variable.
Let 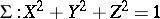 be the unit sphere in the Euclidean
be the unit sphere in the Euclidean  -space, and let
-space, and let 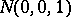 and
and  be its north and south pole, respectively; let
be its north and south pole, respectively; let  and
and  be planes tangent to
be planes tangent to  at the points
at the points  and
and 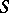 respectively; let
respectively; let 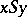 and
and  be coordinate systems in
be coordinate systems in 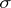 and
and  with axes parallel to the corresponding axes of the system
with axes parallel to the corresponding axes of the system  in the plane
in the plane 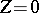 and pointing in the same directions; let
and pointing in the same directions; let  be the stereographic projection of
be the stereographic projection of 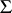 onto
onto  from the centre
from the centre 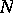 , and let
, and let 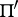 be the stereographic projection of
be the stereographic projection of 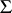 onto
onto  from the centre
from the centre 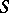 . Then
. Then  is the Bendixson sphere with respect to any one of the planes
is the Bendixson sphere with respect to any one of the planes 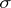 ,
,  . It generates the bijection
. It generates the bijection 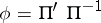 of the plane
of the plane  (punctured at the point
(punctured at the point 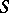 ) onto the plane
) onto the plane  , which is punctured at the point
, which is punctured at the point 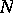 . This bijection is employed in the study of the behaviour of the trajectories of an autonomous system of real algebraic ordinary differential equations of the second order in a neighbourhood of infinity in the phase plane (the right-hand sides of the equations are polynomials of the unknown functions). This bijection reduces the problem to a similar problem in a neighbourhood of the point
. This bijection is employed in the study of the behaviour of the trajectories of an autonomous system of real algebraic ordinary differential equations of the second order in a neighbourhood of infinity in the phase plane (the right-hand sides of the equations are polynomials of the unknown functions). This bijection reduces the problem to a similar problem in a neighbourhood of the point 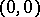 . Named after I. Bendixson.
. Named after I. Bendixson.
References
| [1] | A.A. Andronov, E.A. Leontovich, I.I. Gordon, A.G. Maier, "Qualitative theory of second-order dynamic systems" , Wiley (1973) (Translated from Russian) |
Bendixson sphere. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Bendixson_sphere&oldid=46010