Asymptote
of a curve  with an infinite branch
with an infinite branch
A straight line the distance of which from the point 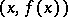 on the curve tends to zero as the point moves along the branch of the curve to infinity. An asymptote can be vertical or inclined. The equation of a vertical asymptote is
on the curve tends to zero as the point moves along the branch of the curve to infinity. An asymptote can be vertical or inclined. The equation of a vertical asymptote is  , where
, where  (
( ) as
) as  (from one side) is satisfied. An inclined asymptote, with equation
(from one side) is satisfied. An inclined asymptote, with equation  , exists if and only if the limits
, exists if and only if the limits
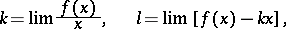 |
exist as  (or as
(or as 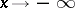 ).
).
Similar formulas are also obtained for parametrized (unbounded) curves in general parametric representation. In polar coordinates an asymptote of a curve  , where
, where 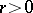 , with slope angle
, with slope angle 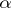 , is defined by the condition
, is defined by the condition  as
as 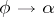 . The distance
. The distance 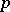 of this asymptote from the coordinate origin is calculated by the formula
of this asymptote from the coordinate origin is calculated by the formula
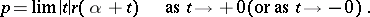 |
If there exists a limit position of the tangent line to the infinite branch of the curve, this position is an asymptote. The converse is not always true. Thus, the curve 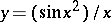 has the asymptote
has the asymptote 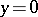 as
as 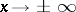 , even though a limit position of the tangent line does not exist. Hyperbolas are the only second-order curves with asymptotes. The asymptotes of the hyperbola
, even though a limit position of the tangent line does not exist. Hyperbolas are the only second-order curves with asymptotes. The asymptotes of the hyperbola 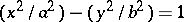 are given by the equations
are given by the equations 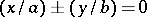 . An inclined asymptote yields a simple (linear with respect to
. An inclined asymptote yields a simple (linear with respect to  ) asymptotic approximation of the function:
) asymptotic approximation of the function:
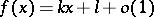 |
as  (or as
(or as  ).
).
References
| [1] | P.K. Rashevskii, "A course of differential geometry" , Moscow (1956) (In Russian) |
| [2] | L.D. Kudryavtsev, "Mathematical analysis" , Moscow (1973) (In Russian) |
Comments
References
| [a1] | A.V. Pogorelov, "Differential geometry" , Noordhoff (1959) (Translated from Russian) |
Asymptote. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Asymptote&oldid=45234