Asymmetry coefficient

The most frequently employed measure of the asymmetry of a distribution, defined by the relationship

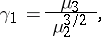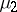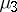where and are the second and third central moments of the distribution, respectively. For distributions that are symmetric with respect to the mathematical expectation, 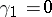; depending on the sign of 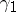one speaks of positive asymmetry (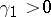) and negative asymmetry (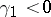). In the case of the binomial distribution corresponding to Bernoulli trials with probability of success ,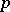

(*)

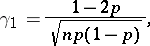one has: If 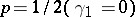, the distribution is symmetric; if 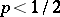or , one obtains typical distribution diagrams with a positive (Fig.a) and negative (Fig.b) asymmetry.

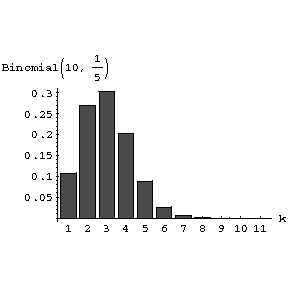
Figure: a013590a

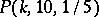. Diagram of the binomial distribution 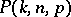corresponding to 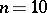Bernoulli trials, with positive asymmetry ().

Figure: a013590b

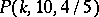. Diagram of the binomial distribution 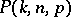corresponding to 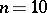Bernoulli trials, with negative asymmetry ().

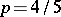The asymmetry coefficient (*) tends to zero as , in accordance with the fact that a normalized binomial distribution converges to the standard normal distribution.

The asymmetry coefficient and the excess coefficient are the most extensively used characteristics of the accuracy with which the distribution function 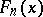of the normalized sum

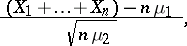where 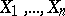are identically distributed and mutually independent with asymmetry coefficient 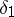, may be approximated by the normal distribution function

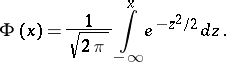Under fairly general conditions the Edgeworth series yields

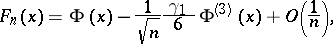where 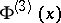is the derivative of order three.

References

[1]	H. Cramér, "Mathematical methods of statistics" , Princeton Univ. Press (1946)
[2]	S.S. Wilks, "Mathematical statistics" , Wiley (1962)

Comments

The asymmetry coefficient is usually called the coefficient of skewness. One correspondingly speaks of the skewness of a distribution and of positive, respectively negative, skewness.

The excess coefficient is more often called the coefficient of kurtosis.