Analytic mapping

analytic morphism

A morphism of analytic spaces considered as ringed spaces (cf. Analytic space; Ringed space). An analytic mapping of a space () into a space () is a pair , where

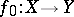is a continuous mapping, while

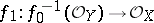is a homomorphism of sheaves of rings on . If the spaces are complex, an analytic mapping is also called a holomorphic mapping.

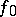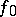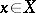If and are reduced analytic spaces, the homomorphism is completely determined by the mapping and is the inverse mapping of the germs of functions corresponding to . Thus, in this case an analytic mapping is a mapping such that for any and for any 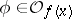one has .

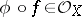A fibre of an analytic mapping

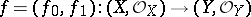at a point is the analytic subspace

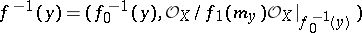of the space 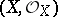, where 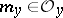is the sheaf of germs of functions that vanish at the point 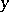. Putting

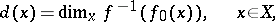one obtains the inequality

(*)

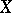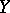If and are reduced complex spaces, then the set

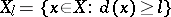is analytic in 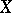for any .

An analytic mapping 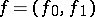is called flat at a point 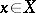if is a flat module over the ring 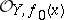. In such a case (*) becomes an equality. An analytic mapping is called flat if it is flat at all points 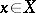. A flat analytic mapping of complex spaces is open. Conversely, if 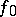is open, 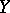is smooth and all fibres are reduced, then 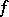is a flat analytic mapping. The set of points of a complex or a rigid analytic space 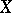at which an analytic mapping 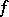is not flat is analytic in 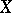. If and are reduced complex spaces, while 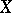has a countable base, then 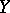contains a dense everywhere-open set over which is a flat analytic mapping. If an analytic mapping

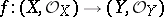of complex spaces is flat, then the set of at which the fibre is not reduced or normal is analytic in .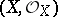

Let 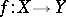be an analytic mapping of reduced complex spaces. If 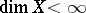, then there exists a stratification

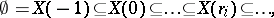where 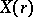are analytic sets and for large , with the following property: Any point 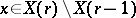has a neighbourhood in such that 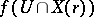is a local analytic set in 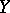, all irreducible components of germs of which have dimension at . If 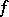is proper, then 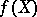is an analytic set in 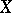. This is a particular case of the finiteness theorem for analytic mappings.

Let 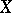, 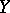be complex spaces and let be compact. Then it is possible to endow the set 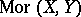of all analytic mappings with the structure of a complex space such that the mapping

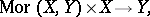which maps the pair 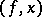into 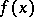, is analytic. In particular, the group of automorphisms of a compact complex space 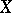is a complex Lie group, acting analytically on .

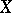References

[1]	R. Remmert, "Projektionen analytischer Mengen" Math. Ann. , 130 (1956) pp. 410–441
[2]	R. Remmert, "Holomorphe und meromorphe Abbildungen komplexer Räume" Math. Ann. , 133 (1957) pp. 328–370
[3]	K. Stein, , Colloquium for topology , Strasbourg (1954)
[4]	J. Frisch, "Points de plattitude d'une morphisme d'espaces analytiques complexes" Invent. Math. , 4 (1967) pp. 118–138
[5]	G. Fisher, "Complex analytic geometry" , Springer (1976)