Analytic curve

analytic arc

A curve in an -dimensional Euclidean space 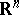, , 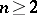which has an analytic parametrization. This means that the coordinates of its points can be expressed as analytic functions of a real parameter 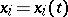, , 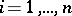, 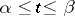i.e. in a certain neighbourhood of each point , , 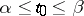the functions can be represented as convergent power series in 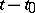, and the derivatives , , 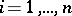do not simultaneously vanish at any point of the segment 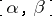. This last condition is sometimes treated separately, and an analytic curve which satisfies it is called a regular analytic curve. An analytic curve is called closed if , .

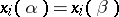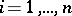An analytic curve in the plane 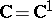of the complex variable 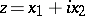can be represented as a complex-analytic function of a real parameter 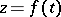, , 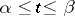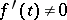on 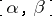. If the analytic curve is located in a domain , then a conformal mapping of 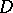into any domain will also yield an analytic curve. If the set of intersection points of two analytic curves is infinite, these analytic curves coincide.

In general, in a complex space 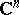, , the complex coordinates 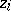of the points of an analytic curve can be represented as analytic functions of a real parameter 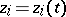, , 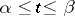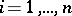. It should be noted, however, that if , the term "analytic curve" may sometimes denote an analytic surface of complex dimension one.

On a Riemann surface an analytic curve 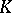can be represented as 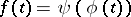, where is a local uniformizing parameter of the points 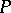on and 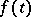is an analytic function of a real parameter in a neighbourhood of any point .

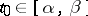References

[1]	A.I. Markushevich, "Theory of functions of a complex variable" , 1 , Chelsea (1977) (Translated from Russian)
[2]	B.V. Shabat, "Introduction of complex analysis" , 1–2 , Moscow (1969) pp. Chapt. 3 (In Russian)

References

[a1]	R.C. Gunning, H. Rossi, "Analytic functions of several complex variables" , Prentice-Hall (1965)