Alternion

A hypercomplex number. Alternions may be considered as a generalization of the complex numbers, double numbers (cf. Double and dual numbers) and quaternions. The algebra of alternions of order and of index 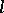is an algebra of dimension 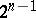over the field of real numbers, with unit element 1 and a system of generators 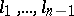, in which the multiplication satisfies the formula

where 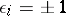, the value 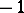occurs 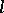times and 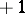occurs 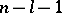times, respectively. A base of the algebra is formed by the unit element and by elements of the form

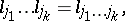where 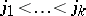. In this base any alternion 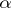can be written as

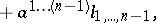where 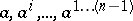are real numbers. The alternion 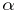conjugate to the alternion 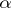is defined by the formula

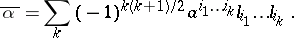The following equalities hold

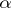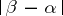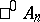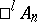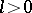The product is always a positive real number; the quantity is called the modulus of the alternion . If the number is taken as the distance between two alternions and , then the algebras and , , are isometric to the Euclidean space 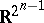and the pseudo-Euclidean spaces 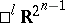, respectively. The algebra 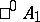is isomorphic to the field of real numbers; 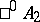is isomorphic to the field of complex numbers; 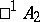is isomorphic to the algebra of double numbers; 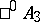is isomorphic to the skew-field of quaternions; and 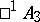and are isomorphic to the so-called algebras of anti-quaternions. The elements of 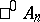are the so-called Clifford numbers. The algebra was studied by P. Dirac in the context of the spin of an electron.

The algebras of alternions are special cases of Clifford algebras (cf. Clifford algebra).

References

[1]	B.A. Rozenfel'd, "Non-Euclidean geometry" , Moscow (1955) (In Russian)