Algebraic polynomial of best approximation

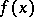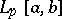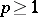A polynomial deviating least from a given function. More precisely, let a measurable function be in () and let 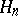be the set of algebraic polynomials of degree not exceeding . The quantity

(*)

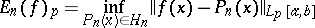is called the best approximation, while a polynomial for which the infimum is attained is known as an algebraic polynomial of best approximation in 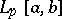. Polynomials which deviate least from a given continuous function in the uniform metric (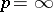) were first encountered in the studies of P.L. Chebyshev (1852), who continued to study them in 1856 [1]. The existence of algebraic polynomials of best approximation was established by E. Borel [2]. Chebyshev proved that 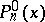is an algebraic polynomial of best approximation in the uniform metric if and only if Chebyshev alternation occurs in the difference 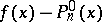; in this case such a polynomial is unique. If 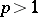, the algebraic polynomial of best approximation is unique due to the strict convexity of the space . If 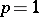, it is not unique, but it has been shown by D. Jackson [3] to be unique for continuous functions. The rate of convergence of to zero is given by Jackson's theorems (cf. Jackson theorem).

In a manner similar to (*) an algebraic polynomial of best approximation is defined for functions in a large number of unknowns, say 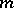. If the number of variables 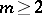, an algebraic polynomial of best approximation in the uniform metric is, in general, not unique.

References

[1]	P.L. Chebyshev, "Questions on smallest quantities connected with the approximate representation of functions (1859)" , Collected works , 2 , Moscow-Leningrad (1947) pp. 478; 152–236 (In Russian)
[2]	E. Borel, "Leçons sur les fonctions de variables réelles et les développements en séries de polynômes" , Gauthier-Villars (1905)
[3]	D. Jackson, "A general class of problems in approximation" Amer. J. Math. , 46 (1924) pp. 215–234
[4]	A.L. Garkavi, "The theory of approximation in normed linear spaces" Itogi Nauk. Mat. Anal. 1967 (1969) pp. 75–132 (In Russian)

Comments

Instead of the long phrase "algebraic polynomial of best approximation" one also uses the shorter phrase "best algebraic approximationbest algebraic approximation" , which is not to be confused with the phrase "best approximation" for the least error .

References

[a1]	N.I. [N.I. Akhiezer] Achiezer, "Theory of approximation" , F. Ungar (1956) (Translated from Russian)
[a2]	E.W. Cheney, "Introduction to approximation theory" , Chelsea, reprint (1982) pp. 203ff
[a3]	G.W. Meinardus, "Approximation von Funktionen und ihre numerische Behandlung" , Springer (1964) pp. Chapt. 1, §5