Action of a group on a manifold

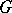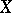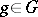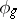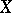The best-studied case of the general concept of the action of a group on a space. A topological group acts on a space if to each there corresponds a homeomorphism of (onto itself) satisfying the following conditions: 1) 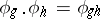; 2) for the unit element 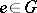the mapping is the identity homeomorphism; and 3) the mapping 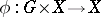, 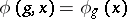is continuous. If 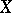and 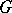have supplementary structures, the actions of 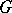which are compatible with such structures are of special interest; thus, if 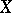is a differentiable manifold and 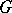is a Lie group, the mapping 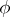is usually assumed to be differentiable.

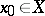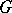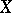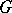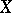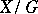The set is called the orbit (trajectory) of the point with respect to the group ; the orbit space is denoted by , and is also called the quotient space of the space with respect to the group . An important example is the case when is a Lie group and is a subgroup; then is the corresponding homogeneous space. Classical examples include the spheres , the Grassmann manifolds 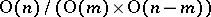, and the Stiefel manifolds 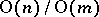(cf. Grassmann manifold; Stiefel manifold). Here, the orbit space is a manifold. This is usually not the case if the action of the group is not free, e.g. if the set 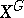of fixed points is non-empty. 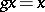A free action of a group is an action for which follows if for any 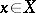. On the contrary, 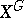is a manifold if 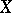is a differentiable manifold and the action of 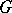is differentiable; this statement is valid for cohomology manifolds ove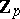r for 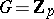as well (Smith's theorem).

If 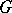is a non-compact group, the space is usually inseparable, and this is why a study of individual trajectories and their mutual locations is of interest. The group 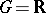of real numbers acting on a differentiable manifold 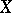in a differentiable manner is a classical example. The study of such dynamical systems, which in terms of local coordinates is equivalent to the study of systems of ordinary differential equations, usually involves analytical methods.

If 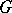is a compact group, it is known that if 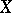is a manifold and if each 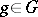, , 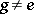acts non-trivially on 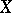(i.e. not according to the law 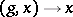), then 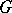is a Lie group [8]. Accordingly, the main interest in the action of a compact group is the action of a Lie group.

Let 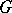be a compact Lie group and let 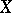be a compact cohomology manifold. The following results are typical. A finite number of orbit types exists in , and the neighbourhoods of an orbit look like a direct product (the slice theorem); the relations between the cohomology structures of the spaces 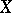, 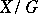and are of interest.

If 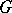is a compact Lie group, a differentiable manifold and if the action

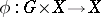is differentiable, then one naturally obtains the following equivalence relation: if and only if it is possible to find an such that the boundary 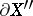has the form 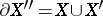and such that 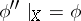, 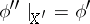. If the group 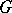acts freely, the equivalence classes can be found from the one-to-one correspondence with the bordisms 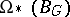of the classifying space (cf. Bordism).

Recent results (mid-1970s) mostly concern: 1) the determination of types of orbits with various supplementary assumptions concerning the group 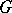and the manifold ([6]); 2) the classification of group actions; and 3) finding connections between global invariants of the manifold 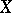and local properties of the group actions of 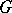in a neighbourhood of fixed points of . In solving these problems an important part is played by: methods of modern differential topology (e.g. surgery methods); 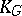-theory [1], which is the analogue of 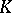-theory for -vector bundles; bordism and cobordism theories [3]; and analytical methods of studying the action of the group 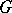based on the study of pseudo-differential operators in 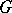-bundles [2], [7].

References

[1]	M.F. Atiyah, "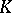-theory: lectures" , Benjamin (1967)
[2]	M.F. Atiyah, I.M. Singer, "The index of elliptic operators" Ann. of Math. (2) , 87 (1968) pp. 484–530
[3]	V.M. Bukhshtaber, A.S. Mishchenko, S.P. Novikov, "Formal groups and their role in the apparatus of algebraic topology" Russian Math. Surveys , 26 (1971) pp. 63–90 Uspekhi Mat. Nauk , 26 : 2 (1971) pp. 131–154
[4]	P.E. Conner, E.E. Floyd, "Differentiable periodic maps" , Springer (1964)
[5]	G. Bredon, "Introduction to compact transformation groups" , Acad. Press (1972)
[6]	W.Y. Hsiang, "Cohomology theory of topological transformation groups" , Springer (1975)
[7]	D.B. Zagier, "Equivariant Pontryagin classes and applications to orbit spaces" , Springer (1972)
[8]	, Proc. conf. transformation groups , Springer (1968)
[9]	, Proc. 2-nd conf. compact transformation groups , Springer (1972)

References

[a1]	T. Petrie, J.D. Randall, "Transformation groups on manifolds" , M. Dekker (1984)