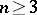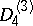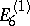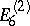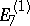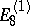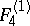Lie algebra, graded
A Lie algebra 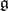 over a field
over a field 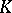 that is graded by means of an Abelian group
that is graded by means of an Abelian group 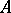 , that is, which splits into a direct sum of subspaces
, that is, which splits into a direct sum of subspaces 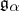 ,
, 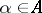 , in such a way that
, in such a way that 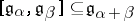 . If
. If 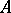 is an ordered group, then for every filtered Lie algebra (cf. Filtered algebra) the graded algebra associated with it is a graded Lie algebra.
is an ordered group, then for every filtered Lie algebra (cf. Filtered algebra) the graded algebra associated with it is a graded Lie algebra.
Graded Lie algebras play an important role in the classification of simple finite-dimensional Lie algebras, Jordan algebras and their generalizations, and primitive pseudo-groups of transformations (see [3], [4]). For any semi-simple real Lie algebra its Cartan decomposition can be regarded as a 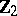 -grading. The local classification of symmetric Riemannian spaces reduces to the classification of
-grading. The local classification of symmetric Riemannian spaces reduces to the classification of 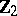 -graded simple complex Lie algebras [6].
-graded simple complex Lie algebras [6].
Some constructions of graded Lie algebras.
1) Let 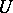 be an associative algebra (cf. Associative rings and algebras) endowed with an increasing filtration
be an associative algebra (cf. Associative rings and algebras) endowed with an increasing filtration 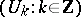 , suppose that
, suppose that 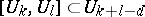 , where
, where 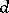 is a fixed natural number, and let
is a fixed natural number, and let 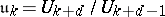 . Then the commutation operation in
. Then the commutation operation in 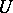 induces in the space
induces in the space 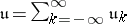 the structure of a
the structure of a  -graded Lie algebra. In this way one can obtain some Lie algebras of functions with the Poisson brackets as commutator. In the next two examples,
-graded Lie algebra. In this way one can obtain some Lie algebras of functions with the Poisson brackets as commutator. In the next two examples, 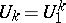 for
for 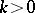 and
and 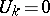 for
for 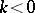 .
.
a) Let 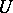 be the algebra of linear differential operators with polynomial coefficients and let
be the algebra of linear differential operators with polynomial coefficients and let 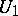 be the subspace spanned by its generators
be the subspace spanned by its generators 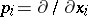 ,
,  ,
, 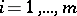 . Then
. Then 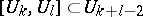 and
and  is the Lie algebra of polynomials in
is the Lie algebra of polynomials in 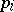 and
and 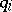 with the usual Poisson brackets.
with the usual Poisson brackets.
b) Let 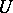 be the universal enveloping algebra of a finite-dimensional Lie algebra
be the universal enveloping algebra of a finite-dimensional Lie algebra 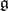 and let
and let 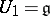 . Then
. Then 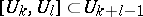 and
and  is canonically isomorphic (as a vector space) to the symmetric algebra over
is canonically isomorphic (as a vector space) to the symmetric algebra over 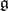 , that is, to the algebra of polynomials on the dual space
, that is, to the algebra of polynomials on the dual space 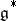 (the Poincaré–Birkhoff–Witt theorem). If
(the Poincaré–Birkhoff–Witt theorem). If 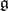 is the Lie algebra of a connected Lie group
is the Lie algebra of a connected Lie group 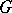 , then the commutator of elements of
, then the commutator of elements of  can be interpreted either as the Poisson brackets for the corresponding left-invariant functions on the cotangent bundle
can be interpreted either as the Poisson brackets for the corresponding left-invariant functions on the cotangent bundle 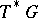 , or as the Poisson brackets on each orbit of the co-adjoint representation, defined by means of the standard symplectic structure on these orbits.
, or as the Poisson brackets on each orbit of the co-adjoint representation, defined by means of the standard symplectic structure on these orbits.
2) Suppose that  and that
and that 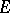 is an
is an  -dimensional vector space over
-dimensional vector space over 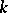 endowed with a non-singular quadratic form
endowed with a non-singular quadratic form 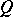 ; let
; let  be an orthogonal basis of
be an orthogonal basis of 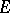 . The decomposition of the Clifford algebra
. The decomposition of the Clifford algebra 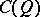 into the sum of one-dimensional subspaces
into the sum of one-dimensional subspaces 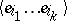 ,
, 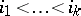 , is a
, is a  -grading of it. For
-grading of it. For 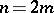 the elements of the algebra
the elements of the algebra 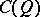 with zero trace form a simple graded Lie algebra of type
with zero trace form a simple graded Lie algebra of type 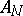 ,
, 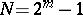 ; its grading has a high degree of symmetry; in particular, all graded subspaces are equivalent. Similar gradings (by means of various finite groups) exist for other simple Lie algebras [1].
; its grading has a high degree of symmetry; in particular, all graded subspaces are equivalent. Similar gradings (by means of various finite groups) exist for other simple Lie algebras [1].
3) To every Lie pseudo-group of transformations corresponds a Lie algebra of vector fields. The germ 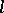 of this Lie algebra at any point has a natural
of this Lie algebra at any point has a natural 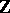 -filtration
-filtration
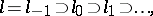 |
where  contains the germs of those vector fields whose coordinates can be expanded in power series without terms of degree less than
contains the germs of those vector fields whose coordinates can be expanded in power series without terms of degree less than  . The associated graded Lie algebra can be interpreted as a Lie algebra of polynomial vector fields.
. The associated graded Lie algebra can be interpreted as a Lie algebra of polynomial vector fields.
The classification of simple graded Lie algebras.
To simple primitive Lie pseudo-groups correspond the following four series of simple infinite-dimensional graded Lie algebras (see [5]):
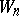 , the Lie algebra of all polynomial vector fields in the
, the Lie algebra of all polynomial vector fields in the  -dimensional affine space;
-dimensional affine space;
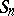 , its subalgebra consisting of vector fields with zero divergence;
, its subalgebra consisting of vector fields with zero divergence;
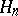 , where
, where 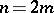 , the subalgebra consisting of vector fields that annihilate the differential form
, the subalgebra consisting of vector fields that annihilate the differential form
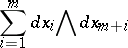 |
(Hamiltonian vector fields);
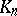 , where
, where 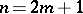 , the subalgebra consisting of vector fields that multiply the differential form
, the subalgebra consisting of vector fields that multiply the differential form
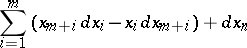 |
by a function.
Over fields of characteristic  one can define simple finite-dimensional graded Lie algebras analogous to
one can define simple finite-dimensional graded Lie algebras analogous to  ,
, 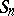 ,
, 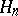 , and
, and  (see [5]).
(see [5]).
Simple graded Lie algebras of another type are obtained in the following way [4]. Let 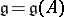 be the Lie algebra defined by means of an indecomposable Cartan matrix
be the Lie algebra defined by means of an indecomposable Cartan matrix 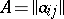 ,
, 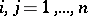 (from now on the notation of the article Cartan matrix is used). The algebra
(from now on the notation of the article Cartan matrix is used). The algebra 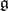 is endowed with a
is endowed with a 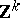 -grading so that
-grading so that 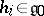 ,
, 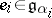 ,
,  , where
, where 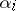 is the row
is the row 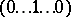 with
with  in the
in the 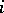 -th place. Elements
-th place. Elements  for which
for which 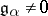 are called roots, and the
are called roots, and the 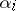 are called simple roots. Any root is a linear combination of simple roots with integer coefficients of the same sign and
are called simple roots. Any root is a linear combination of simple roots with integer coefficients of the same sign and 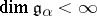 for any
for any  . The quotient algebra
. The quotient algebra  of
of 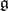 with respect to its centre, which lies in
with respect to its centre, which lies in 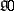 , is simple as a graded algebra, that is, it does not have non-trivial graded ideals.
, is simple as a graded algebra, that is, it does not have non-trivial graded ideals.
Let 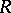 be the totality of linear combinations of rows of the matrix
be the totality of linear combinations of rows of the matrix 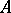 with positive coefficients. Then one of the following cases holds:
with positive coefficients. Then one of the following cases holds:
(P) 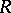 contains a row all elements of which are positive;
contains a row all elements of which are positive;
(Z) 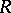 contains a zero row;
contains a zero row;
(N)  contains a row all elements of which are negative.
contains a row all elements of which are negative.
In the case (P),  is a simple finite-dimensional Lie algebra. In the case (N),
is a simple finite-dimensional Lie algebra. In the case (N), 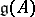 is a simple infinite-dimensional Lie algebra. In the case (Z), the algebra
is a simple infinite-dimensional Lie algebra. In the case (Z), the algebra 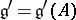 is simple only as a graded algebra. It can be converted in a
is simple only as a graded algebra. It can be converted in a 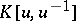 -algebra so that: a)
-algebra so that: a) 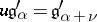 , where
, where  is a row of positive numbers; and b) the quotient algebra
is a row of positive numbers; and b) the quotient algebra 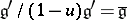 is a simple finite-dimensional Lie algebra. The greatest common divisor of all components
is a simple finite-dimensional Lie algebra. The greatest common divisor of all components 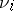 of the row
of the row  , which is equal to 1, 2 or 3, is called the index of the algebra
, which is equal to 1, 2 or 3, is called the index of the algebra 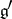 .
.
The following table is a list of all simple graded Lie algebras with Cartan matrix of type (Z). Here the algebra 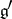 is denoted by the same symbol as the associated simple finite-dimensional Lie algebra
is denoted by the same symbol as the associated simple finite-dimensional Lie algebra 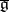 , but with the addition of its index, given in brackets.
, but with the addition of its index, given in brackets.
The diagram of simple roots describes the matrix 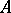 . Its vertices correspond to the simple roots; the
. Its vertices correspond to the simple roots; the 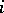 -th and
-th and 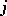 -th vertices are joined by an
-th vertices are joined by an 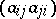 -multiple edge, directed from the
-multiple edge, directed from the 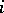 -th vertex to the
-th vertex to the 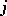 -th if
-th if 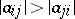 , and undirected if
, and undirected if 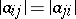 . Above the vertices stand the numbers
. Above the vertices stand the numbers 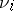 .'
.'
<tbody> </tbody>
|
By means of graded Lie algebras with Cartan matrix of type (Z) one can classify 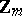 -graded simple finite-dimensional Lie algebras (see [4], [2]). Namely, let
-graded simple finite-dimensional Lie algebras (see [4], [2]). Namely, let  , where
, where 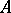 satisfies condition (Z), and let
satisfies condition (Z), and let 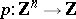 be a homomorphism such that
be a homomorphism such that 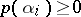 and
and  . Then
. Then 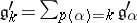 for any
for any 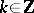 is mapped isomorphically onto the subspace
is mapped isomorphically onto the subspace 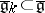 , which depends only on the residue of
, which depends only on the residue of 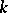 modulo
modulo 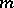 , and the decomposition
, and the decomposition 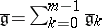 is a
is a 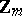 -grading of
-grading of 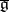 . If the field
. If the field 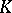 is algebraically closed, then by the method described one obtains, without repetition, all
is algebraically closed, then by the method described one obtains, without repetition, all  -graded simple finite-dimensional Lie algebras over
-graded simple finite-dimensional Lie algebras over 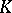 . The index of
. The index of 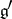 is equal to the order of the automorphism
is equal to the order of the automorphism  ,
, 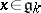 , of the algebra
, of the algebra 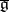 modulo the group of inner automorphisms.
modulo the group of inner automorphisms.
There is a classification of simple 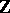 -graded Lie algebras
-graded Lie algebras 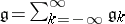 satisfying the conditions: a)
satisfying the conditions: a) 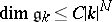 for some
for some 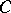 and
and 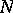 ; b)
; b) 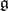 is generated by the subspace
is generated by the subspace 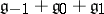 ; and c) the representation of
; and c) the representation of 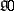 on
on  is irreducible. In this case either
is irreducible. In this case either 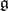 is finite-dimensional or it is one of the algebras
is finite-dimensional or it is one of the algebras 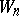 ,
, 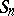 ,
, 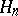 ,
,  , or it is the algebra
, or it is the algebra  defined by a Cartan matrix of type (Z), endowed with a suitable
defined by a Cartan matrix of type (Z), endowed with a suitable  -grading [4].
-grading [4].
A Lie superalgebra is sometimes called a 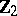 -graded Lie algebra.
-graded Lie algebra.
References
| [1] | A.V. Alekseevskii, "Finite commutative Jordan subgroups of complex simple Lie groups" Funct. Anal. Appl. , 8 : 4 (1974) pp. 277–279 Funktsional. Anal. Prilozhen. , 8 : 4 (1974) pp. 1–4 |
| [2] | E.B. Vinberg, "The Weyl group of a graded Lie algebra" Math. USSR Izv. , 10 (1976) pp. 436–496 Izv. Akad. Nauk SSSR Ser. Mat. , 40 : 3 (1976) pp. 488–526 |
| [3] | I.L. Kantor, "Certain generalizations of Jordan algebras" Trudy Sem. Vektor. Tenzor. Anal. , 16 (1972) pp. 407–499 (In Russian) |
| [4] | V.G. Kac, "Simple irreducible graded Lie algebras of finite growth" Math. USSR Izv. , 2 (1968) pp. 1271–1312 Izv. Akad. Nauk SSSR Ser. Mat. , 32 : 6 (1968) pp. 1323–1367 |
| [5] | A.I. Kostrikin, I.R. Shafarevich, "Graded Lie algebras of finite characteristic" Math. USSR Izv. , 3 (1969) pp. 237–304 Izv. Akad. Nauk SSSR Ser. Mat. , 33 : 2 (1969) pp. 252–322 |
| [6] | S. Helgason, "Differential geometry, Lie groups, and symmetric spaces" , Acad. Press (1978) |
Comments
The Lie algebras 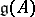 are called Kac–Moody algebras; they have close connections with many areas of mathematics and mathematical physics (cf. [a2]).
are called Kac–Moody algebras; they have close connections with many areas of mathematics and mathematical physics (cf. [a2]).
There is a second notion which also sometimes goes by the name of graded Lie algebra. This is a 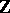 - or
- or 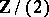 -graded vector space
-graded vector space 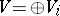 with a multiplication
with a multiplication
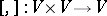 |
such that
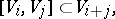 |
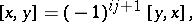 |
for all 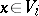 ,
, 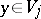 , and
, and
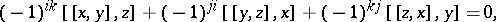 |
for all  ,
, 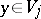 ,
, 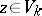 . One also says that
. One also says that 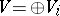 has been equipped with a graded Lie product or graded Lie bracket.
has been equipped with a graded Lie product or graded Lie bracket.
Graded Lie brackets naturally arise, for instance, in cohomology theory in the context of deformations of algebras and complex structures, [a4]. A graded vector space 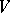 with a graded Lie bracket is not a Lie algebra in the usual sense of the word. A Lie superalgebra is a
with a graded Lie bracket is not a Lie algebra in the usual sense of the word. A Lie superalgebra is a 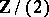 -graded vector space with a
-graded vector space with a 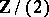 -graded Lie bracket.
-graded Lie bracket.
Of fundamental importance in recent progress in quantum field theory is the Virasoro algebra. This is a (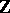 -graded) Lie algebra with a basis
-graded) Lie algebra with a basis 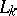 (
(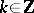 ) and
) and  , and the following commutation relations:
, and the following commutation relations:
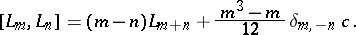 |
See [a1].
References
| [a1] | V.G. Kac, A.K. Raina, "Bombay lectures on highest weight representations" , World Sci. (1987) |
| [a2] | V.G. Kac, "Infinite-dimensional Lie algebras" , Cambridge Univ. Press (1985) |
| [a3] | O. Mathieu, "Classification des algèbres de Lie graduées simples de croissance 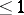 " Invent. Math. , 86 (1986) pp. 371–426 " Invent. Math. , 86 (1986) pp. 371–426 |
| [a4] | M. Hazewinkel (ed.) M. Gerstenhaber (ed.) , Deformation theory of algebras and structures and applications , Kluwer (1988) |
Lie algebra, graded. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lie_algebra,_graded&oldid=44947
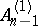 ,
, 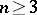
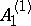
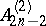 ,
, 
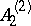
 ,
, 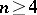
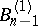 ,
, 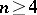
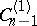 ,
, 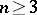
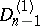 ,
, 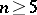
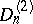 ,
,