Extremal set

A collection of solutions of the Euler equation, depending on arbitrary constants and filling without mutual intersections some part of the -dimensional space. Here is the number of unknown functions , , on which the functional to be minimized,

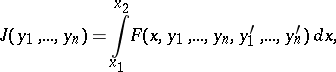depends. Euler's equation is understood in the vector sense, that is, it is a system of ordinary differential equations of the second order:

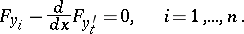Two methods for constructing an extremal set are indicated below.

Let the object of investigation be a pencil of extremals emanating from a given point in an 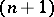-dimensional space. If the extremals of the pencil do not intersect each other in some neighbourhood of 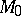(except at ), then they form an extremal set (a central extremal set) in this neighbourhood.

Another method of constructing extremals consists in constructing the set of extremals that are transversal to a surface 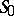given in the -dimensional space by an equation

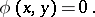If at every point of this surface the transversality conditions

in total, determine the value of the derivatives 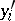, , then by taking these values as initial values of the derivatives one can draw through a point of 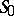an extremal that intersects transversally. If in a neighbourhood of this surface the above extremals do not intersect each other, then they form an extremal set (an ordinary, or proper extremal set).

The construction of an extremal set is the starting point in the discussion of questions connected with the construction of a field of extremals (cf. Extremal). An extremal set is an extremal field if there is a one-parameter family of surfaces that intersect the extremals of the set transversally.

References

[1]	V.I. Smirnov, "A course of higher mathematics" , 4 , Addison-Wesley (1964) (Translated from Russian)

References

[a1]	I.M. Gel'fand, S.V. Fomin, "Calculus of variations" , Prentice-Hall (1963) (Translated from Russian)