Dupin indicatrix

curvature indicatrix

A plane curve illustrating the normal curvatures of a surface at a point of this surface. The Dupin indicatrix lies in the tangent plane to the surface at the point and there it is described by the radius vector of length , where is the normal curvature of at in the direction . Let be a parametrization of 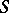in a neighbourhood of 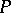. One introduces a coordinate system on the tangent plane to at 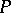, taking 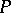as the coordinate origin, and the vectors 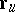and 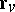as the basis vectors of this coordinate system. The equation of the Dupin indicatrix will then be

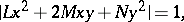where and are the coordinates of a point on the Dupin indicatrix, and , 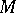and are the coefficients of the second fundamental form of 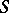calculated at . The Dupin indicatrix is: a) an ellipse if is an elliptic point (a circle if 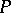is an umbilical point); b) a pair of conjugate hyperbolas if 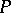is a hyperbolic point; and c) a pair of parallel straight lines if 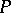is a parabolic point. The curve is named after Ch. Dupin (1813), who was the first to use this curve in the study of surfaces.

Figure: d034180a

References

[1]	V.F. Kagan, "Foundations of the theory of surfaces in a tensor setting" , 2 , Moscow-Leningrad (1948) (In Russian)

Comments

The Dupin indicatrix does not exist at a flat point.

The Dupin indicatrix at 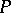can be obtained as the limit of suitably normalized (inter)sections of the surface with planes parallel to the tangent plane of at which are approaching this plane, see [a1], p. 370; [a2], p. 363-365.

References

[a1]	M. Berger, B. Gostiaux, "Differential geometry: manifolds, curves, and surfaces" , Springer (1988) (Translated from French)
[a2]	H.S.M. Coxeter, "Introduction to geometry" , Wiley (1961)
[a3]	C.C. Hsiung, "A first course in differential geometry" , Wiley (1981) pp. Chapt. 3, Sect. 4