Tensor algebra
A part of tensor calculus in which algebraic operations on tensors (cf. Tensor on a vector space) are studied.
The tensor algebra of a unitary module 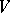 over a commutative associative ring
over a commutative associative ring 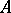 with unit is the algebra
with unit is the algebra  over
over 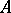 whose underlying module has the form
whose underlying module has the form
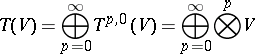 |
and in which multiplication is defined with the help of tensor multiplication (cf. Tensor on a vector space). Besides the contravariant tensor algebra, the covariant tensor algebra
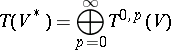 |
is also considered, as well as the mixed tensor algebra
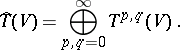 |
If the module 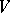 is free and finitely generated, then
is free and finitely generated, then 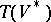 is naturally isomorphic to the algebra of all multilinear forms (cf. Multilinear form) on
is naturally isomorphic to the algebra of all multilinear forms (cf. Multilinear form) on 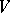 . Any homomorphism
. Any homomorphism  of
of 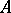 -modules naturally defines a tensor algebra homomorphism
-modules naturally defines a tensor algebra homomorphism 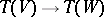 .
.
The tensor algebra 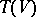 is associative, but in general not commutative. Its unit is the unit of the ring
is associative, but in general not commutative. Its unit is the unit of the ring 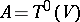 . Any
. Any  -linear mapping of the module
-linear mapping of the module 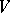 into an associative
into an associative 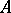 -algebra
-algebra 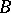 with a unit can be naturally extended to a homomorphism of algebras
with a unit can be naturally extended to a homomorphism of algebras 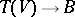 mapping the unit to the unit. If
mapping the unit to the unit. If 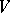 is a free module with basis
is a free module with basis 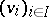 , then
, then  is the free associative algebra with system of generators
is the free associative algebra with system of generators 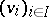 .
.
References
| [1] | N. Bourbaki, "Elements of mathematics. Algebra: Algebraic structures. Linear algebra" , 1 , Addison-Wesley (1974) pp. Chapt.1;2 (Translated from French) |
| [2] | A.I. Kostrikin, Yu.I. Manin, "Linear algebra and geometry" , Gordon & Breach (1989) (Translated from Russian) |
Tensor algebra. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Tensor_algebra&oldid=43389