Bicyclic semi-group

The semi-group with unit element and two generators 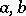subject to the single generating relation 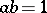. One of the realizations of the bicyclic semi-group is the Cartesian square 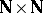, where 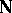is the set of non-negative integers, with respect to the operation

The bicyclic semi-group is an inversion semi-group and as such is monogenic, i.e. is generated by a single element. The idempotents (cf. Idempotent) of the bicyclic semi-group form a chain, which is ordered with respect to the type of the positive numbers. The bicyclic semi-group is bisimple (cf. Simple semi-group).

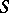The bicyclic semi-group often occurs in theoretical investigations concerning semi-groups, not only as a representative of certain important classes of semi-groups, but also as a "block" , which defines the structure of individual semi-groups. Thus, for any idempotent of a -simple, but not completely -simple semi-group there exists a bicyclic sub-semi-group in containing as the unit element (cf. [1], Para. 2.7). The elements and 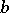of the bicyclic semi-group defined as above, are, respectively, its left and right multiplying elements (i.e. there exist proper subsets 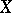and in such that , 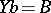). Moreover, in a semi-group 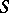with unit element the element will be a left multiplier if and only if 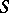contains the bicyclic semi-group whose unit element is identical with ; a similar theorem is also valid for right multiplying elements, so that 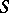has left multiplying elements if and only if it also has right multiplying elements.

References

[1]	A.H. Clifford, G.B. Preston, "Algebraic theory of semi-groups" , 1–2 , Amer. Math. Soc. (1961–1967)
[2]	E.S. Lyapin, "Semigroups" , Amer. Math. Soc. (1974) (Translated from Russian)